You probably learned some time ago that chemical bonds are what hold atoms together to form the more complicated aggregates that we know as molecules and extended solids. Chemists talk about bonds all the time, and draw pictures of them as lines joining atom symbols. Teachers often identify them as the little sticks that connect the spheres that represent atoms in a plastic molecular model. So it's not surprising that we sometimes tend to think of chemical bonds as “things”. But no one has ever seen a chemical bond, and there is no reason to believe that they really even exist as physical objects.
"SOMETIMES IT SEEMS to me that a bond between two atoms has become so real, so tangible, so friendly, that I can almost see it. Then I awake with a little shock, for a chemical bond is not a real thing. It does not exist. No one has ever seen one. No one ever can. It is a figment of our own imagination."
C.A. Coulson (1910-1974) was an English theoretical chemist who played a central role in the development of quantum theories of chemical bonding.
It is probably more useful to regard a chemical bond as an effect that causes certain atoms to join together to form enduring structures that have unique physical and chemical properties.
So although the "chemical bond" (as a physical object) may be no more than a convenient fiction, chemical bonding, which leads to the near-infinity of substances (31 million in mid-2007), lies at the very core of chemistry.
The forces that hold bonded atoms together are basically just the same kinds of electrostatic attractions that bind the electrons of an atom to its positively-charged nucleus;
are simultaneously attracted to two nuclei.
This is the most important fact about chemical bonding that you should know, but it is not of itself a workable theory of bonding because it does not describe the conditions under which bonding occurs, nor does it make useful predictions about the properties of the bonded atoms.
Our views of what constitutes chemical bonding are still evolving, according to a 2007 article in Chemical and Engineering News(85 37-40). This "buckyball-and-mitt" synthesized in 2007 by Andrzej Sygula is a case in point. The buckyball C60 resides in the C60H28"buckybowl". There are no traditional "chemical bonds" between the ball and the mit!
image from C&EN 85 (13) 2008
Most people think of molecules as the particles that result when atoms become joined together in some way. This conveys the general picture, but a somewhat better definition that we will use in these lessons is
A more restrictive definition distinguishes between a "true" molecule that exists as an independent particle, and an extended solid that can only be represented by its simplest formula. Methane, CH4, is an example of the former, while sodium chloride, which does not contain any discrete NaCl units, is the most widely-known extended solid. But because we want to look at chemical bonding in the most general way, we will avoid making this distinction here except in a few special cases. In order to emphasize this "aggregate of atoms" definition, we will often use terms such as "chemical species" and "structures" in place of "molecules" in this lesson.
The definition written above is an operational one; that is, it depends on our ability to observe and measure the molecule's properties. Clearly, this means that the molecule must retain its identity for a period of time long enough to carry out these observations. For most of the molecules of chemical interest, this presents no difficulty. But it does happen that some structures that we can write formulas for, such as He2, have such brief lives that no significant properties have been observed. So to some extent, what we consider to be a molecule depends on the technology we use to observe them, and this will necessarily change with time.
Structure, structure, structure!
And what are those properties that characterize a particular kind of molecule and distinguish it from others? Just as real estate is valued by "location, location, location", the identity of a chemical species is defined by its structure. In its most fundamental sense, the structure of a molecule is specified by the identity of its constituent atoms and the sequence in which they are joined together, that is, by the bonding connectivity. This, in turn, defines the bonding geometry— the spatial relationship between the bonded atoms.
Structures without molecules: stability and reactivity
The precise definition of bonding energy is described in another lesson and is not important here. For the moment you only need to know that in any stable structure, the potential energy of its atoms is lower than that of the individual isolated atoms. Thus the formation of methane from its gaseous atoms (a reaction that cannot be observed under ordinary conditions but for which the energetics are known from indirect evidence)
4 H(g) + C(g) → CH4
is accompanied by the release of heat, and is thus an exothermic process. The quantity of heat released is related to the stability of the molecule. The smaller the amount of energy released, the more easily can the molecule absorb thermal energy from the environment, driving the above reaction in reverse and leading to the molecule's decomposition. A highly stable molecule such as methane must be subjected to temperatures of more than 1000°C for significant decomposition to occur. But the noble-gas molecule KrF2 is so weakly bound that it decomposes even at 0°C, and the structure He2 has never been observed. If a particular arrangement of atoms is too unstable to reveal its properties at any achievable temperature, then it does not qualify to be called a molecule.
There are many molecules that are energetically stable enough to meet the above criterion, but are so reactive that their lifetimes are too brief to make their observation possible. The molecule CH3, methyl, is a good example: it can be formed by electrical discharge in gaseous CH4, but it is so reactive that it combines with almost any molecule it strikes (even another CH3) within a few collisions. It was not until the development of spectroscopic methods (in which a molecule is characterized by the wavelengths of light that it absorbs or emits) that methyl was recognized as a stable albeit shamelessly promiscuous molecule that is an important intermediate in many chemical processes ranging from flames to atmospheric chemistry.
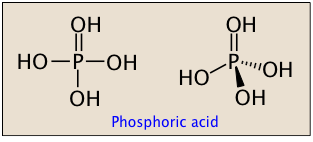Chemical species are traditionally represented by structural formulas such as the ones for phosphoric acid, H3PO4, which we show here. The lines, of course, represent the "chemical bonds" of the molecule.
More importantly, the structural formula of a molecule defines its connectivity, as was illustrated in the comparison of ethanol and dimethyl ether shown in the previous section.
One limitation of such formulas is that they are drawn on a two-dimensional surface, whereas most molecules have a three-dimensional shape.
The wedge-shaped lines in the structural formula on the right are one way of indicating which bonds extend above or below the viewing plane, providing a kind of pseudo-3D view. You will probably be spared having to learn this convention until you get into Organic Chemistry.
Three-dimensional models (either real plastic ones or images that incorporate perspective and shading) reveal much more about a molecule's structure. The ball-and-stick and space-filling renditions are widely employed, but each has its limitations, as seen in the following examples that compare different ways of depicting the structures of the same two molecules:
Simple structural formulas in two dimensions show the molecule's connectivity, but nothing more.
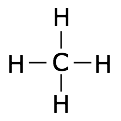
↑ This simple structural formula of methane, CH4, projects its 3-dimentional structure onto a 2D surface.
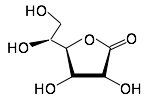
↑ The structural formula of ascorbic acid (vitamin C) is commonly enhanced with wedge-shaped bonds to show that they extend above the plane of the paper or screen.
Ball-and-stick models show 3-dimensional views of the "chemical bonds" and their geometry. although with the individual atoms unrealisticly separated.
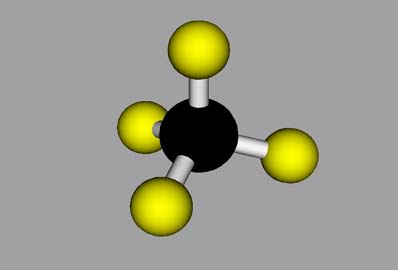
This image correctly expresses the tetrahedral coordination of the four C—H bonds.
Ascorbic acid
Space-filling models don't attempt to depict the bonds, but show the relative sizes of the atoms and general shape of the molecule, at the expense of hiding some of the atoms.
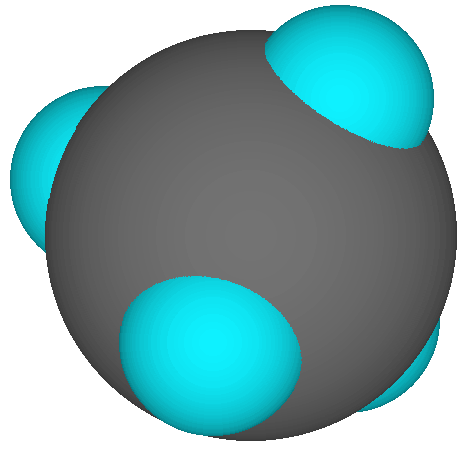
Note how this shows CH4 to be roughly spherical.
[U. Alberta ]
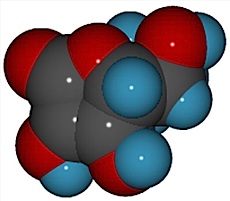
Ascorbic acid
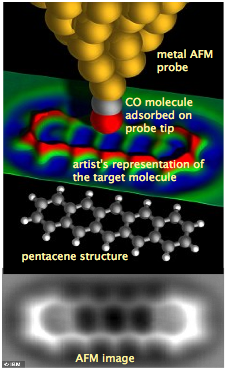Finally, we get to see one! In 2009, IBM scientists in Switzerland succeeded in imaging a real molecule, using a technique known as atomic force microscopy in which an atoms-thin metallic probe is drawn ever-so-slightly above the surface of an immobilized pentacene molecule cooled to nearly absolute zero. In order to improve the image quality, a molecule of carbon monoxide was placed on the end of the probe.
The image produced by the AFM probe is shown at the very bottom. What is actually being imaged is the surface of the electron clouds of the molecule, which consists of five fused hexagonal rings of carbon atoms with hydrogens on its periphery. The tiny bumps that correspond to these hydrogen atoms attest to the remarkable resolution of this experiment.
The original article was published in Science magazine; see here for an understandable account of this historic work.
Molecular modeling and visualization
The purpose of rendering a molecular structure in a particular way is not to achieve "realism" (whatever that might be), but rather to convey useful information of some kind. Modern computer rendering software takes its basic data from various kinds of standard structural databases which are compiled either from experimental X-ray scattering data, or are calculated from theory.
[image]
Example: Caffeine
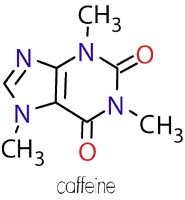Caffeine's molecular formula, C8H10N4O2, tells us its composition, but conveys no information about how how its 24 atoms are connected, and thus how it differs from any number of other compounds having the same formula. In order to reveal its connectivity, we use its structural formula.
Although the structure at the right does not look like
the one on the cup, their connectivities are identical,
so the two structures are equivalent.
As an exercise,
see if you are able to confirm this.
For most "ordinary" chemistry, the structural formula is all we need. Suppose, however, that you wish to understand more about how caffeine exerts its stimulating effect on the body. Like many drugs, caffeine binds to specific sites on proteins; the efficacy of this binding is usually highly dependent on the both the shape of the drug and the manner in which electric charge is distributed over this shape.
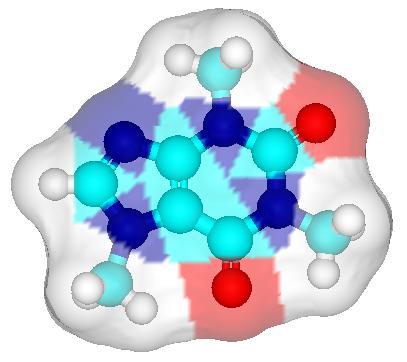In this context, it is important to understand that the outer "surface" of a molecule is defined by the veil of negative charge that originates in the valence electrons of the atoms but which tends to be spread over the entire molecule to a distance that can significantly affect van der Waals interactions with neighboring molecules.
For this purpose, one employs a mixture of chemical intuition and molecular modeling computer software to generate an image such as the one shown here.
In this depiction of Caffeine, the atoms are coded by color: white=H, red=O, light blue=C, dark blue=N.
Example: Sucrose
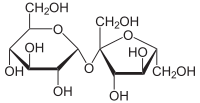
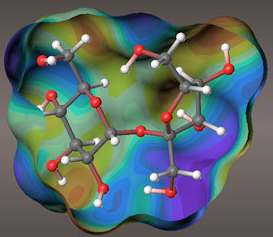Sucrose — ordinary "sugar" — occurs naturally in fruits and vegetables, as well as in everyone's kitchen and in all too many foods and beverages. Its structural formula shows that it is really a "double sugar" (a disaccharide) in which two monosaccharides, glucose and fructose, are joined together.
The overall shape and distribution of electric charge at the surface enable it to bind to the sweetness receptors on the tongue, and — more importantly to the enzyme that catalyzes the hydrolysis reaction that breaks the sucrose into its two monosaccharide components, releasing the glucose which fuels our body's cells.
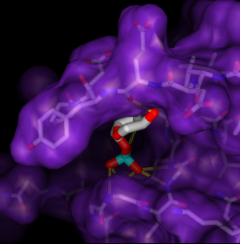
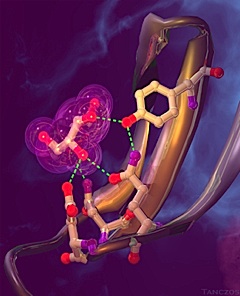Knowing the properties of molecular surfaces is vitally important to understanding any process that depends on one molecule remaining in physical contact with another. Catalysis is one example, but one of the main interests at the present time is biological signalling, in which a relatively small molecule binds to or "docks" with a receptor site on a much larger one, often a protein. Sophisticated molecular modeling software such as was used to produce these images is now a major tool in many areas of research biology.
[Images: left, right ]
Visualizing very large molecules such as carbohydrates and proteins that may contain tens of thousands of atoms presents obvious problems. The usual technique is to simplify parts of the molecule, representing major kinds of extended structural units by shapes such as ribbons or tubes which are twisted or bent to approximate their conformations. These are then gathered to reveal the geometrical relations of the various units within the overall structure. Individual atoms, if shown at all, are restricted to those of special interest.
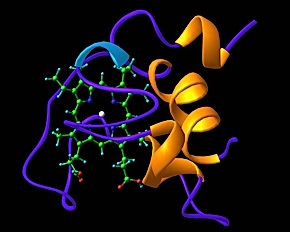 A cytochrome heme-binding protein composed of five peptide helices (gold and blue). A heme molecule lodged in its binding site is shown in green, with the central Fe(II) ion in white. Cytochromes are essential elements of respiratory electron transfer. [source] |
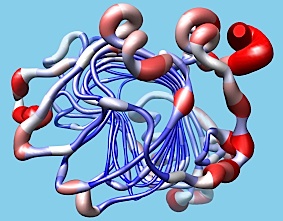 This worm-like creature is as evil as it looks; it is a highly nuanced rendering of the enzyme pectate lyase that is involved in the rotting of plant tissue. [source] |
|
Some of these images can stand as artistic creations in their own right. This seems to be especially true of those that render special aspects of molecular surfaces; the two on the left below could well be mistaken for paintings by Jean Miró and Salvador Dalí, respectively.
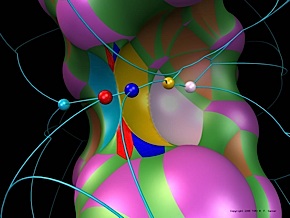 Computational chemist Michel Sanner has developed special software for exploring the boundary between a molecule and the solvent molecules which surround it. This image uses colored spheres to denote points at which the "solvent-excluded surface" intersects with itself. |
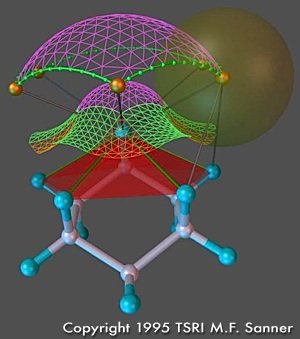 This creature making its graceful descent illustrates some of the topological forms involved in molecular surface problems. This image and the one at the left are reproduced here with the kind permission of Prof. Sanner. |
[image from the Swiss Proteomics site] |
Study of the surface properties of large molecules is crucial for understanding how proteins, carbohydrates, and DNA interact with smaller molcules, especially those involved in transport of ions and small molecule across cell membranes, immune-system behavior, and signal transduction processes such as the "turning on" of genes.
See here for links to a wide variety of sources relating to visualization and molecular modeling.
When we talk about the properties of a particular chemical bond, we are really discussing the relationship between two adjacent atoms that are part of the molecule. Diatomic molecules are of course the easiest to study, and the information we derive from them helps us interpret various kinds of experiments we carry out on more complicated molecules.
It is important to bear in mind that the exact properties of a specific kind of bond will be determined in part by the nature of the other bonds in the molecule; thus the energy and length of the C–H bond will be somewhat dependent on what other atoms are connected to the carbon atom. Similarly, the C-H bond length can vary by as much a 4 percent between different molecules. For this reason, the values listed in tables of bond energy and bond length are usually averages taken over a variety of compounds that contain a specific atom pair..
In some cases, such as C—O and C—C, the variations can be much greater, approaching 20 percent. In these cases, the values fall into groups which we interpret as representative of single- and multiple bonds: double, and triple.
Potential energies of bonded atoms
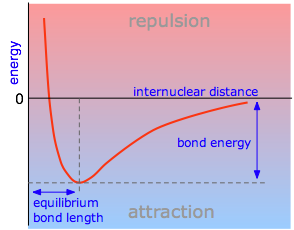The energy of a system of two atoms depends on the distance between them. At large distances the energy is zero, meaning “no interaction”. At distances of several atomic diameters attractive forces dominate, whereas at very close approaches the force is repulsive, causing the energy to rise. The attractive and repulsive effects are balanced at the minimum point in the curve. Plots that illustrate this relationship are known as Morse curves, and they are quite useful in defining certain properties of a chemical bond.
The internuclear distance at which the potential energy minimum occurs defines the bond length. This is more correctly known as the equilibrium bond length, because thermal motion causes the two atoms to vibrate about this distance. In general, the stronger the bond, the smaller will be the bond length.
Attractive forces operate between all atoms, but unless the potential energy minimum is at least of the order of RT, the two atoms will not be able to withstand the disruptive influence of thermal energy long enough to result in an identifiable molecule. Thus we can say that a chemical bond exists between the two atoms in H2. The weak attraction between argon atoms does not allow Ar2 to exist as a molecule, but it does give rise to the van Der Waals force that holds argon atoms together in its liquid and solid forms.
Potential energy and kinetic energy Quantum theory tells us that an electron in an atom possesses kinetic energy K as well as potential energy P, so the total energy E is always the sum of the two: E = P + K. The relation between them is surprisingly simple: K = –0.5 P. This means that when a chemical bond forms (an exothermic process with ΔE < 0), the decrease in potential energy is accompanied by an increase in the kinetic energy (embodied in the momentum of the bonding electrons), but the magnitude of the latter change is only half as much, so the change in potential energy always dominates. The bond energy –ΔE has half the magnitude of the fall in potential energy.
Energies of chemical bonds
The bond energy is the amount of work that must be done to pull two atoms completely apart; in other words, it is the same as the depth of the “well” in the potential energy curve shown above. This is almost, but not quite the same as the bond dissociation energy actually required to break the chemical bond; the difference is the very small zero-point energy.
This relationship will be clarified below in the section on bond vibrational frequencies.
How bond energies are measured
Bond energies are usually determined indirectly from thermodynamic data, but there are two main experimental ways of measuring them directly:
1. The direct thermochemical methodinvolves separating the two atoms by an electrical discharge or some other means, and then measuring the heat given off when they recombine. Thus the energy of the C—C single bond can be estimated from the heat of the recombination reaction between methyl radicals, yielding ethane:
CH3 + CH3 → H3C–CH3
Although this method is simple in principle, it is not easy to carry out experimentally. The highly reactive components must be prepared in high purity and in a stream of moving gas.
2. The spectroscopic methodis based on the principle that absorption of light whose wavelength corresponds to the bond energy will often lead to the breaking of the bond and dissociation of the molecule. For some bonds, this light falls into the green and blue regions of the spectrum, but for most bonds ultraviolet light is required. The experiment is carried out by observing the absorption of light by the substance being studied as the wavelength is decreased. When the wavelength is sufficiently small to break the bond, a characteristic change in the absorption pattern is observed.
Spectroscopy is quite easily carried out and can yield highly precise results, but this method is only applicable to a relatively small number of simple molecules. The major problem is that the light must first be absorbed by the molecule, and relatively few molecules happen to absorb light of a wavelength that corresponds to a bond energy.
Experiments carried out on diatomic molecules such as O2 and CS yield unambiguous values of bond energy, but for more complex molecules there are complications. For example, the heat given off in the CH3 combination reaction written above will also include a small component that represents the differences in the energies of the C-H bonds in methyl and in ethane. These can be corrected for by experimental data on reactions such as
CH3 + H → CH4
CH2 + H → CH3
By assembling a large amount of experimental information of this kind, a consistent set of average bond energies can be obtained (see table below.) The energies of double bonds are greater than those of single bonds, and those of triple bonds are higher still.
Bond energies and heats of reaction
See here for more on heats of reaction and their significance.
One can often get a very good idea of how much heat will be absorbed or given off in a reaction by simply finding the difference in the total bond energies contained in the reactants and products. The strength of an individual bond such as O–H depends to some extent on its environment in a molecule (that is, in this example, on what other atom is connected to the oxygen atom), but tables of "average" energies of the various common bond types are widely available and can provide useful estimates of the quantity of heat absorbed or released in many chemical reaction.
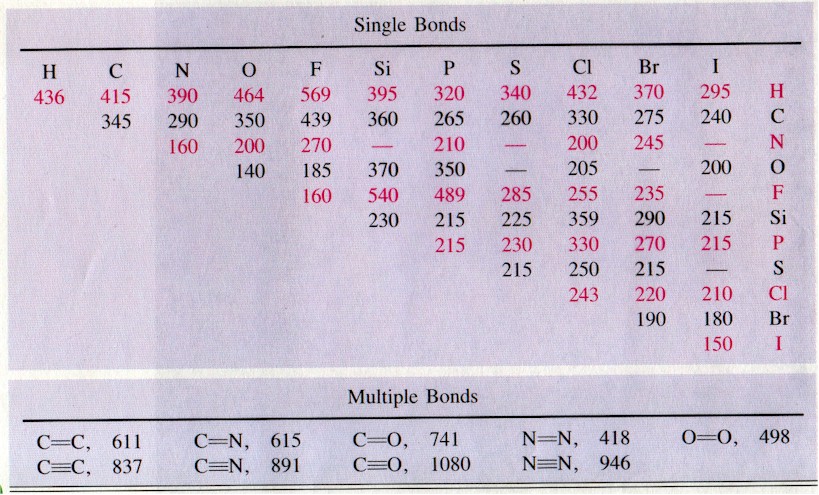
The bond energy units in the above table are kilojoules per mole.
As an example, consider the reaction of chlorine with methane to produce dichloromethane and hydrogen chloride:
CH4(g) + 2 Cl2(g) → CH2Cl2(g) + 2 HCl(g)
In this reaction, two C–Cl bonds and two H–Cl bonds are broken, and two new C–Cl and H–Cl bonds are formed. The net change associated with the reaction is
2(C–H) + 2(Cl–Cl) – 2(C–Cl) – 2(H–Cl) = (830 + 486 –660 – 864) kJ
which comes to –208 kJ per mole of methane; this agrees quite well with the observed heat of reaction, which is –202 kJ/mol.
Lengths of chemical bonds
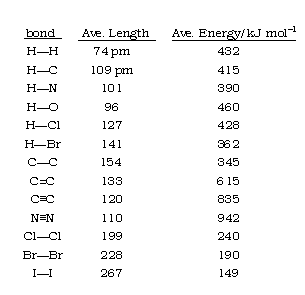The length of a chemical bond the distance between the centers of the two bonded atoms (the internuclear distance.) Bond lengths have traditionally been expressed in Ångstrom units, but picometers are now preferred (1Å = 10-8 cm = 100 pm.) Bond lengths are typically in the range 1-2 Å or 100-200 pm. Even though the bond is vibrating, equilibrium bond lengths can be determined experimentally to within ±1 pm.
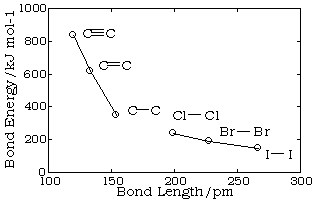Bond lengths depend mainly on the sizes of the atoms, and secondarily on the bond strengths, the stronger bonds tending to be shorter. Bonds involving hydrogen can be quite short; The shortest bond of all, H–H, is only 74 pm. Multiply-bonded atoms are closer together than singly-bonded ones; this is a major criterion for experimentally determining the multiplicity of a bond. This trend is clearly evident in the above plot which depicts the sequence of carbon-carbon single, double, and triple bonds.
The most common method of measuring bond lengths in solids is by analysis of the diffraction or scattering of X-rays when they pass through the regularly-spaced atoms in the crystal. For gaseous molecules, neutron- or electron-diffraction can also be used.
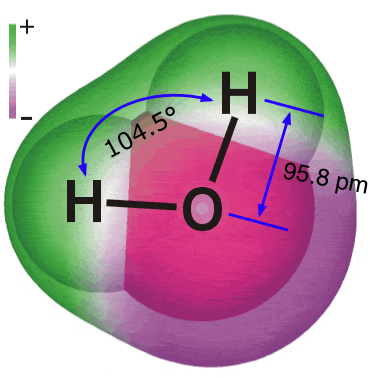The complete structure of a molecule requires a specification of the coordinates of each of its atoms in three-dimensional space. This data can then be used by computer programs to construct visualizations of the molecule as discussed above. One such visualization of the water molecule, with bond distances and the HOH bond angle superimposed on a space-filling model, is shown here. (It is taken from an excellent reference source on water). The colors show the results of calculations that depict the way in which electron charge is distributed around the three nuclei.
Bond stretching and infrared absorption
When an atom is displaced from its equilibrium position in a molecule, it is subject to a restoring force which increases with the displacement. A spring follows the same law (Hooke’s law); a chemical bond is therefore formally similar to a spring that has weights (atoms) attached to its two ends. A mechanical system of this kind possesses a natural vibrational frequency which depends on the masses of the weights and the stiffness of the spring. These vibrations are initiated by the thermal energy of the surroundings; chemically-bonded atoms are never at rest at temperatures above absolute zero.
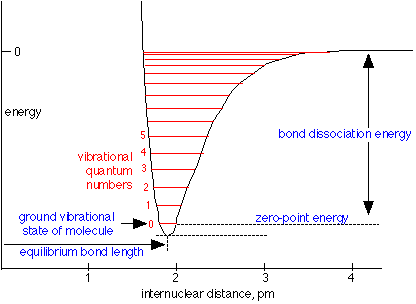On the atomic scale in which all motions are quantized, a vibrating system can possess a series of vibrational frequencies, or states. These are depicted by the horizontal lines in the potential energy curve shown here. Notice that the very bottom of the curve does not correspond to an allowed state because at this point the positions of the atoms are precisely specified, which would violate the uncertainty principle. The lowest-allowed, or ground vibrational state is the one denoted by 0, and it is normally the only state that is significantly populated in most molecules at room temperature. In order to jump to a higher state, the molecule must absorb a photon whose energy is equal to the distance between the two states.
For ordinary chemical bonds, the energy differences beween these natural vibrational frequencies correspond to those of infrared light. Each wavelength of infrared light that excites the vibrational motion of a particular bond will be absorbed by the molecule. In general, the stronger the bond and the lighter the atoms it connects, the higher will be its natural stretching frequency and the shorter the wavelength of light absorbed by it. Studies on a wide variety of molecules have made it possible to determine the wavelengths absorbed by each kind of bond (See here for a brief list.) By plotting the degree of absorption as a function of wavelength, one obtains the infrared spectrum of the molecule which allows one to "see" what kinds of bonds are present.
Infrared spectrum of alcohol The low points in the plot below indicate the frequencies of infrared light that are absorbed by ethanol (ethyl alcohol),
CH3CH2OH. Notice how stretching frequencies involving hydrogen are higher, reflecting the smaller mass of that atom. Only the most prominent absorption bands are noted here.
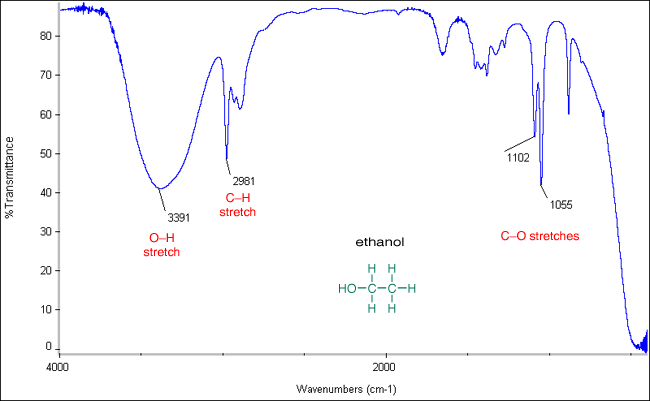
Actual infrared spectra are complicated by the presence of more complex motions (stretches involving more than two atoms, wagging, etc.), and absorption to higher quantum states (overtones), so infrared spectra can become quite complex. This is not necessarily a disadvantage, however, because such spectra can serve as a "fingerprint" that is unique to a particular molecule and can be helpful in identifying it. Largely for this reason, infrared spectrometers are standard equipment in most chemistry laboratories.
Infrared absorption and the greenhouse effect
The aspect of bond stretching and bending frequencies that impacts our lives most directly is the way that some of the gases of the atmosphere absorb infrared light and thus affect the heat balance of the Earth. Owing to their symmetrical shapes, the principal atmospheric components N2 and O2 do not absorb infrared light, but the minor components water vapor and carbon dioxide are strong absorbers, especially in the long-wavelength region of the infrared. Absorption of infrared light by a gas causes its temperature to rise, so any source of infrared light will tend to warm the atmosphere; this phenomenon is known as the greenhouse effect.
The incoming radiation from the Sun (which contains relatively little long-wave infrared light) passes freely through the atmosphere and is absorbed by the Earth's surface, warming it up and causing it to re-emit some of this energy as long-wavelength infrared. Most of the latter is absorbed by the H2O and CO2 , the major greenhouse gasis in the unpolluted atmosphere, effectively trapping the radiation as heat. Thus the atmosphere is heated by the Earth, rather than by direct sunlight. Without the “greenhouse gases” in the atmosphere, the Earth's heat would be radiated away into space, and our planet would be too cold for life.
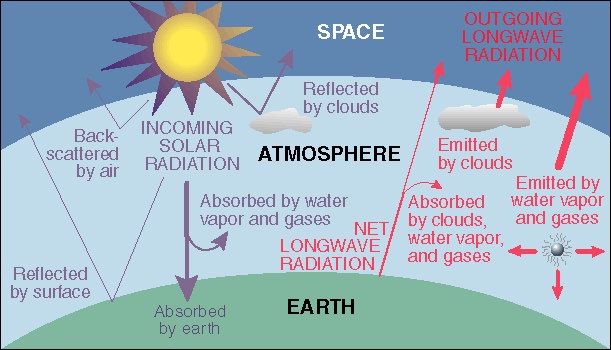
Radiation balance of the Earth In order to maintain a constant average temperature, the quantity of radiation (sunlight) absorbed by the surface must be exactly balanced by the quantity of long-wavelength infrared emitted by the surface and atmosphere and radiated back into space. Atmospheric gases that absorb this infrared light (depicted in red on the right part of this diagram) partially block this emission and become warmer, raising the Earth's temperature. This diagram is from the U. of Oregon Web page referenced below.
Since the beginning of the Industrial Revolution in the 19th century, huge quantities of additional greenhouse gases have been accumulating in the atmosphere. Carbon dioxide from fossil fuel combustion has been the principal source, but intensive agriculture also contributes significant quantities of methane (CH4) and nitrous oxide (N2O) which are also efficient far-infrared absorbers. The measurable increase in these gases is believed by many to be responsible for the increase in the average temperature of the Earth that has been noted over the past 50 years— a trend that could initiate widespread flooding and other disasters if it continues.
Links on the greenhouse effect and global warming:
University of Oregon Global Warming page
U.S. EPA Global Warming site
Make sure you thoroughly understand the following essential concepts that have been presented above.
- How would you define a chemical bond?
- What is meant by the connectivity of a molecule? What additional information might be needed in order to specify its structure?
- Explain the difference between stability and reactivity, and how these factors might prevent a given structure from existing long enough to qualify as a molecule.
- Sketch out a potential energy curve for a typical diatomic molecule, and show how it illustrates the bond energy and bond length.
- Explain how the heat released or absorbed in a chemical reaction can be related to the bond energies of the reactants and products.
- State the major factors that determine the distance between two bonded atoms.
- Describe, in a general way, how the infrared spectrum of a substance can reveal details about its molecular structure.
