Note: this document will print in an appropriately modified format (9 pages)

On this page:

This page presents examples that cover most of the kinds of equilibrium problems you are likely to encounter in a first-year university course.

Reading this page will not teach you how to work equilibrium problems! The only one who can teach you how to interpret, understand, and solve problems is yourself.

So don't just "read" this and think you are finished. You need to find and solve similar problems on your own. Look over the problems in your homework assignment or at the end of the appropriate chapter in a textbook, and see how they fit into the general types described below. When you can solve them without looking at the examples below, you will be well on your way!

[Rasmus Grønfeldt]

1 Measuring and calculating equilibrium constants

Clearly, if the concentrations or pressures of all the components of a reaction are known, then the value of K can be found by simple substitution. Observing individual concentrations or partial pressures directly may be not always be practical, however. If one of the components is colored, the extent to which it absorbs light of an appropriate wavelength may serve as an index of its concentration. Pressure measurements are ordinarily able to measure gonly the total pressure of a gaseous mixture, so if two or more gaseous products are present in the equilibrium mixture, the partial pressure of one may need to be inferred from that of the other, taking into account the stoichiometry of the reaction.

Problem Example 2

Ordinary white phosphorus, P₄, forms a vapor which dissociates into diatomic molecules at high temperatures: P₄(g)→ 2 P₂(g)

A sample of white phosphorus, when heated to 1000°C, formed a vapor having a total pressure of 0.20 atm and a density of 0.152 g L^–1. Use this information to evaluate the equilibrium constant K_p for this reaction.

Solution: The first question you should ask yourself is what does the observed density of the equilibrium mixture have to do with K_p? The answer: it provides information about the equilibrium composition, which we require in order to calculate K_p. But first, begin in the usual way by laying out the information required to express K_p in terms of an unknown x.

	P₄	2 P₂
initial moles:	1	0	Since K is independent of the number of moles, assume the simplest case.
moles at equilibrium:	1 - x	2x	x is the fraction of P₄ that dissociates.
eq. mole fractions:			The denominator is the total number of moles: (1-x) + 2x = 1+ x.
eq. partial pressures:			Partial pressure is the mole fraction times the total pressure.

Expressing the equilibrium constant in terms of x gives

Yes, this is a quadratic, but not one you need to solve, so don't pan!c! All we need to do is use the density information to determine what fraction x of P₄ is dissociated, and then substitute it into the equation to find the value of K_p .

At this point we hope you remember those gas laws that you were told you would be using later in the course! The density of a gas is directly proportional to its molecular weight, so you need to calculate the densities of pure P₄ and pure P₂ vapors under the conditions of the experiment. The observed density of the equilibrium mixture, 0.152 gL^–1 will be somewhere in between the densities of the two pure gases, so you can find the dissociation fraction x by simple proportion..

The molecular weight of phosphorus is 31.97, giving a molar mass of 127.9 g for P₄. This mass must be divided by the volume to find the density; assuming ideal gas behavior, the volume of 127.9 g (1 mole) of P₄ is given by RT/P, which works out to 522 L (remember to use the absolute temperature here.) The density of pure P₄ vapor under the conditions of the experiment is then

d = m/V = (128 g mol_^–1) × = (522 L mol^–1) = 0.245 g L^–1.

The density of pure P₂ would be half this, or 0.122 g L^–1.

The difference between these two limiting densities is 0.123 g L^–1, and the difference between the density of pure P₄ and that of the equilibrium mixture is (.245 –.152) g L^–1 = 0.093 g L^–1. The fraction x of P₄ that is dissociated is given by the ratio 0.093 ÷ 0.123 = 0.76. Substituting this into the equilibrium expression above gives K_p = 16.

Comment: The moral of this story is that you should always be ready to make use of simple proportionality when appropriate. And in doing so, sketching out a simple diagram as show here can often help.

2 Predicting equilibrium concentrations

This is by far the most common kind of equilibrium problem you will encounter: starting with an arbitrary number of moles of each component, how many moles of each will be present when the system comes to equilibrium?

The principal source of confusion and error for beginners relates to the need to determine the values of several unknowns (a concentration or pressure for each component) from a single equation, the equilibrium expression. The key to this is to make use of the stoichiometric relationships between the various components, which usually allow us to express the equilibrium composition in terms of a single variable. The easiest and most error-free way of doing this is adopt a systematic approach in which you create and fill in a small table as shown in the following problem example. You then substitute the equilibrium values into the equilibrium constant expression, and solve it for the unknown.

This very often involves solving a quadratic or higher-order equation. Quadratics can of course be solved by using the familiar quadratic formula, but it is often easier to use an algebraic or graphical approximation, and for higher-order equations this is the only practical approach. There is almost never any need to get an exact answer, since the equilibrium constants you start with are rarely known all that precisely anyway.

Problem Example 3

Phosgene (COCl₂) is a poisonous gas that dissociates at high temperature into two other poisonous gases, carbon monoxide and chlorine. The equilibrium constant
K_p = 0.0041 at 600°K. Find the equilibrium composition of the system after 0.124 atm of COCl₂ is allowed to reach equilibrium at this temperature.

Solution: Start by drawing up a table showing the relationships between the components:

	COCl₂	CO	Cl₂
initial pressures:	0.124 atm		0
change:	–x	+x	+x>
equilibrium pressures:	0.124 – x	x	x

Substitution of the equilibrium pressures into the equilibrium expression gives

This expression can be rearranged into standard polynomial form
x² +.0041 x – 0.00054 = 0 and solved by the quadratic formula, but we will simply obtain an approximate solution by iteration. Because the equilibrium constant is small, we know that x will be rather small compared to 0.124, so the above relation can be approximated by

which gives x = 0.0225. To see how good this is, substitute this value of x into the denominator of the original equation and solve again:

This time, solving for x gives 0.0204. Iterating once more, we get

and x = 0.0206 which is sufficiently close to the previous to be considered the final result. The final partial pressures are then 0.104 atm for COCl₂, and 0.0206 atm each for CO and Cl₂.

Comment: using the quadratic formula to find the exact solution yields the two roots –0.0247 (which we ignore) and 0.0206, which show that our approximation is quite good.

Problem Example 4

The gas-phase dissociation of phosphorus pentachloride to the trichloride has Kp = 3.60 at 540°C:

PCl₅ → PCl₃ + Cl₂

What will be the partial pressures of all three components if 0.200 mole of PCl₅ and 3.00 moles of PCl₃ are combined and brought to equilibrium at this temperature and at a total pressure of 1.00 atm?

Solution: As always, set up a table showing what you know (first two rows) and then expressing the equilibrium quantities:

	PCl₅	PCl₃	Cl₂
initial moles:	0.200	3.00	0
change:	–x	+x	+x
equilibrium moles:	0.200 – x	3.00 + x	x
eq. partial pressures:

The partial pressures in the bottom row were found by multiplying the mole fraction of each gas by the total pressure: P_i = X_iP_t. The term in the denominator of each mole fraction is the total number of moles of gas present at equilibrium: (0.200 – x) + (3.00 + x) + x = 3.20 + x.
Substituting the equilibrium partial pressures into the equilibrium expression, we have

whose polynomial form is 4.60 x² + 13.80 x – 2.304 = 0.

Plotting this on a graphical calculator yields x = 0.159 as the positive root:

Substitution of this root into the expressions for the equilibrium partial pressures in the table yields the following values: P(PCl₅) = 0.012 atm, P(PCl₃) = 0.94 atm,
P(Cl₂) = 0.047 atm.

3 Effects of dilution on equilibrium

In the section that introduced the LeChâtelier principle, it was mentioned that diluting a weak acid such as acetic acid CH₃COOH (“HAc”) will shift the dissociation equilibrium to the right:

HAc + H₂O → H₃O⁺ + Ac_^–

Thus a 0.10M solution of acetic acid is 1.3% ionized, while in a 0.01M solution, 4.3% of the HAc molecules will be dissociated. This is because as the solution becomes more dilute, the product [H₃O⁺][Ac^–] decreases more rapidly than does the [HAc] term. At the same time the concentration of H₂O becomes greater, but because it is so large to start with (about 55.5M), any effect this might have is negligible, which is why no [H₂O] term appears in the equilibrium expression.

For a reaction such as CH₃COOH(l) + C₂H₅OH(l) → CH₃COOC₂H₅(l) + H₂O(l) (in which the water concentration does change), dilution will have no effect on the equilibrium; the situation is analogous to the way the pressure dependence of a gas-phase reaction depends on the number of moles of gaseous components on either side of the equation.

Problem Example 5

The biochemical formation of a disaccharide (double) sugar from two monosaccharides is exemplified by the reaction

fructose + glucose-6-phosphate → sucrose-6-phosphate

(Sucrose is ordinary table sugar.) To what volume should a solution containing 0.050 mol of each monosaccharide be diluted in order to bring about 5% conversion to sucrose phosphate?

Solution: The initial and final numbers of moles are as follows:

	fructose	glucose-6-P	sucrose-6-P
initial moles:	0.05	0.05	0<
equilibrium moles:	0.0485	0.0485	0.0015

Substituting into the expression for K_c in which the solution volume is the unknown, we have

Solving for V gives a final solution volume of 78 mL.

4 Phase distribution equilibria

It often happens that two immiscible liquid phases are in contact, one of which contains a solute. How will the solute tend to distribute itself between the two phases? One’s first thought might be that some of the solute will migrate from one phase into the other until it is distributed equally between the two phases, since this would correspond to the maximum dispersion (randomness) of the solute. This, however, does not take into the account the differing solubilities the solute might have in the two liquids; if such a difference does exist, the solute will preferentially migrate into the phase in which it is more soluble.

For a solute S distributed between two phases a and b the process S_a = S_b is defined by the distribution law

in which K_a,b is the distribution ratio (also called the distribution coefficient) and [S]_i is the solubility of the solute in the phase.

The transport of substances between different phases is of immense importance in such diverse fields as pharmacology and environmental science. For example, if a drug is to pass from the aqueous phase with the stomach into the bloodstream, it must pass through the lipid (oil-like) phase of the epithelial cells that line the digestive tract. Similarly, a pollutant such as a pesticide residue that is more soluble in oil than in water will be preferentially taken up and retained by marine organisms, especially fish, whose bodies contain more oil-like substances; this is basically the mechanism whereby such residues as DDT can undergo biomagnification as they become more concentrated at higher levels within the food chain. For this reason, environmental regulations now require that oil-water distribution ratios be established for any new chemical likely to find its way into natural waters. The standard “oil” phase that is almost universally used is octanol, C₈H₁₇OH. (Examples)

Extraction

Extraction is the chemist's term for using an immiscible solvent to recover a desired product from a liquid solution or solid material.

In preparative chemistry it is frequently necessary to recover a desired product present in a reaction mixture by extracting it into another liquid in which it is more soluble than the unwanted substances. On the laboratory scale this operation is carried out in a separatory funnel as shown here. [image: ransirimal]

Continuous extraction: the Soxhlet extractor

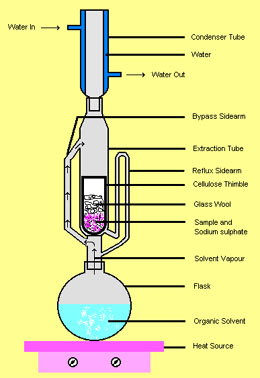An ingenious apparatus invented by Franz von Soxhlet in 1879 has saved laboratory workers untold hours of drudgery by arranging a siphon ("bypass sidearm" in the illustration) to repeatedly send a quantity of solvent through the sample contained in a porous cellulose thimble.

The samples are usually natural products such as plant materials from which something is to be extracted and concentrated. If the desired product is water-soluble, water is used as the extracting solvent; otherwise, an organic solvent is used. Once the appropriate water- or organic fraction is concentrated, the desired component can be isolated by other means.

The beauty of this glass-blower's delight is that the same initial quantity of solvent is re-used. When the solvent is boiled in the flask a the bottom, the hot vapors pass through the "bypass sidearm" up into the condenser at the top, where the condensed liquid then drips down into the reflux column and fills the "extraction tube". When the solvent level level of the upper bend of the "reflux sidearm", it starts a siphon that completely empties the extraction tube, returning the now-enriched solvent to the boiling flask.

This Wikipedia article has an excellent animation of the Soxhlet extractor.

[image]

Problem Example 6

The distribution ratio for iodine between water and carbon disulfide is 650. Calculate 0the concentration of I₂ remaining in the aqueous phase after 50.0 mL of 0.10M I₂ in water is shaken with 10.0 mL of CS₂.

Solution:

The equilibrium constant is

Let m₁ and m₂ represent the numbers of millimoles of solute in the water and CS₂ layers, respectively. K_d can then be written as (m₂/10 mL) ÷ (m₁/50 mL) = 650. The number of moles of solute is (50 mL) × (0.10 mmol mL^–1) = 5.00 mmol, and mass conservation requires that m₁ + m₂ = 5.00 mmol, so m₂ = (5.00 – m₁) mmol and we now have only the single unknown m₁. The equilibrium constant then becomes

((5.00 – m₁) mmol / 10 mL) ÷ (m₁ mmol / 50 mL) = 650.

Simplifying and solving for m₁ yields (0.50 – 0.1)m₁ / (0.02 m₁) = 650, with m₁ = 0.0382 mmol. The concentration of solute in the water layer is (0.0382 mmol) / (50 mL) = 0.000763 M, showing that almost all of the iodine has moved into the CS₂ layer.

Summary

The six Problem Examples presented above were carefully selected to span the range of problem types that students enrolled in first-year college chemistry courses are expected to be able to deal with. If you are able to reproduce these solutions on your own, you should be well prepared on this topic.

The first step in the solution of all but the simplest equilibrium problems is to sketch out a table showing for each component the initial concentration or pressure, the change in this quantity (for example, +2x), and the equilibrium values (for example, .0036 + 2x). In doing so, the sequence of calculations required to get to the answer usually becomes apparent.
Equilibrium calculations often involve quadratic- or higher-order equations. Because concentrations, pressures, and equilibrium constants are seldom known to a precision of more than a few significant figures, there is no need to seek exact solutions. Iterative approximations (as in Problem Example 3) or use of a graphical calculator (Problem Example 4) are adequate and convenient.
Phase distribution equilibria play an important role in chemical separation processes on both laboratory and industrial scales. They are also involved in the movement of chemicals between different parts of the environment, and in the bioconcentration of pollutants in the food chain.

Concept Map