Weak acid-base calculations

On this page:

1 Weak acids and bases in water

"Concentration of acid" and [HA]
Equilibrium concentrations of acid and conjugate base
Degree of dissociation

2 Doing acid/base calculations

Before you start...
pH of a monoprotic acid solution
Working with quadratic equations
Solutions of salts
Exact numerical calculations

3 Solutions of polyprotic acids

Polyprotic acids you should know
Polyprotic acids in pure water
Simplified calculations
Sulfuric acid: a special case

4 Amino acid and Zwitterions

Glycine: the simplest amino acid

What you should be able to do

Most acids are weak; there are hundreds of thousands of them, whereas there are fewer than a dozen strong acids. We can treat weak acid solutions in much the same general way as we did for strong acids. The only difference is that we must now take into account the incomplete "dissociation"of the acid.

We will start with the simple case of the pure acid in water, and then go from there to the more general one in which salts of the acid are present. The latter mixtures are known as buffer solutions and are extremely important in chemistry, physiology, industry and in the environment.

In order to keep our notation as simple as possible, we will refer to “hydrogen ions” and [H⁺] for brevity, and, wherever it is practical to do so, will assume that the acid HA "ionizes" or "dissociates" into H⁺ and its conjugate base A−.

1 Aqueous solutions of weak acids or bases

A weak acid (represented here as HA) is one in which the reaction

HA → A^– + H⁺(1-1)

is incomplete. This means that if we add 1 mole of the pure acid HA to water and make the total volume 1 L, the equilibrium concentration of the conjugate base A^– will be smaller (often much smaller) than 1 M/L, while that of undissociated HA will be only slightly less than 1 M/L.

What does the "concentration of the acid" mean?

The above equation tells us that dissociation of a weak acid HA in pure water yields identical concentrations of its conjugate species. Let us represent these concentrations by x. Then, in our "1 M " solution, the concentration of each species is as shown here:

(1-2)

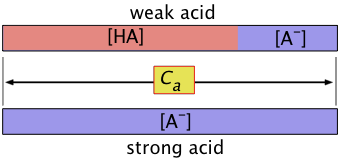
"Concentration of the acid" and [HA] are not the same

When dealing with problems involving acids or bases, bear in mind that when we speak of "the concentration", we usually mean the nominal or analytical concentration which is commonly denoted by C_a.

For a solution made by combining 0.10 mol of pure formic acid HCOOH with sufficient water to make up a volume of 1.0 L, C_a = 0.10 M. But we know that the concentration of the actual species [HCOOH] will be smaller the 0.10 M because some it ends up as the formate ion HCOO^–. It will, of course, always be the case that the sum [HCOOH] + [HCOO^–] = C_a .

For the general case of an acid HA, we can write a mass balance equation

C_a = [HA] + [A^–](1-3)

which reminds us the "A" part of the acid must always be somewhere!

For a strong acid such as hydrochloric, its total dissociation means that
[HCl] = 0, so the mass balance reduces to the trivial expression C_a = [Cl-].
Any acid for which [HA] > 0 is by definition a weak acid.

Similarly, for a base B we can write

C_b = [B] + [HB⁺](1-4)

Equilibrium concentrations of the acid and its conjugate base

*This will be true as long as the acid is not so weak or dilute that we can neglect the small quantity of H⁺ contributed by the autoprotolysis of H₂O.

According to the above equations, the equilibrium concentrations of A^– and H⁺ will be identical*. Let us represent these concentrations by x. Then, in a solution containing 1 M/L of a weak acid, the concentration of each species is as shown here:

(1-5)

Substituting these values into the equilibrium expression for this reaction, we obtain

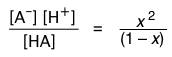(1-6)

In order to predict the pH of this solution, we must solve for x. The presence of terms in both x and x ² here tells us that this is a quadratic equation. But don't panic! In most practical cases in which K_a is 10^–4 or smaller, we can assume that x is much smaller than 1 M, allowing us to make the simplifying approximation

(1 – x) ≈ 1 (1-7)

so that x² ≈ K_a and thus x = √K_a. (1-8)

This approximation will not generally be valid when the acid is very weak or very dilute — But more on this later.

Solutions of arbitrary concentration

The above development was for a solution made by taking 1 mole of acid and adding sufficient water to make its volume 1.0 L. In such a solution, the nominal concentration of the acid, denoted by C_a, is 1 M.

We can easily generalize this to solutions in which C_a has any value:

(1-9)

The above relation is known as a "mass balance on A". It expresses the simple fact that the "A" part of the acid must always be somewhere — either attached to the hydrogen, or in the form of the hydrated anion A^–.

The corresponding equilibrium expression is

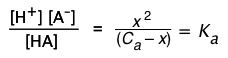(1-10)

and the approximations (when justified) 1-3a and 1-3b become

(C_a – x) ≈ C_a (1-11)

x ≈ (C_a K_a)^½(1-12)

Problem Example 1 - approximate pH of an acetic acid solution

Estimate the pH of a 0.20 M solution of acetic acid, K_a = 1.8 × 10^–5.

Solution: For brevity, we will represent acetic acid CH₃COOH as HAc, and the acetate ion by Ac^–. As before, we set x = [H⁺] = [Ac^–], neglecting the tiny quantity of H⁺ that comes from the dissociation of water.

Substitution into the equilibrium expression yields

The rather small value of K_a suggests that we can drop the x term in the denominator, so that (x² / 0.20) ≈ 1.8E-5 or x ≈ (0.20 × 1.8E–5)^½ = 1.9E-3 M

The pH of the solution is –log (1.9E–3) = 2.7

Degree of dissociation

Even though we know that the process HA → H⁺ + A^– does not correctly describe the transfer of a proton to H₂O, chemists still find it convenient to use the term "ionization" or "dissociation".

The "degree of dissociation" (denoted by(alpha) of a weak acid is just the fraction

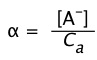(1-13)

which is often expressed as a per cent ( × 100).

Degree of dissociation depends on the concentration

It's important to understand that whereas K_a for a given acid is essentially a constant, \alpha will depend on the concentration of the acid.

Note that these equations are also valid for weak bases if K_b and C_b are used in place of K_a and C_a.

This can be shown by substituting Eq 5 into the expression for K_a:

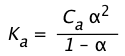(1-14)

Solving this forresults in a quadratic equation, but if the acid is sufficiently weak that we can say (1 – ) ≈ 1, the above relation becomes

(1-15)

the amount of HA that dissociates varies inversely with the square root of the concentration; as C_a approaches zero,
approaches unity and [HA] approaches C_a.

This principle is an instance of the Ostwald dilution law which relates the dissociation constant of a weak electrolyte (in this case, a weak acid), its degree of dissociation, and the concentration.

A common, but incorrect explanation of this law in terms of the Le Châtelier principle states that dilution increases the concentration of water in the equation HA + H₂O → H₃O⁺ + A^–, thereby causing this equilibrium to shift to the right. The error here is that [H₂O] in most aqueous solutions is so large (55.5 M) that it can be considered constant; this is the reason the [H₂O] term does not appear in the expression for K_a. Another common explanation is that dilution reduces [H₃O⁺] and [A^–], thus shifting the dissociation process to the right. But dilution similarly reduces [HA], which would shift the process to the left. In fact, these two processes compete, but the former has greater effect because two species are involved.

It is probably more satisfactory to avoid Le Châtelier-type arguments altogether, and regard the dilution law as an entropy effect, a consequence of the greater dispersal of thermal energy throughout the system. This energy is carried by the molecular units within the solution; dissociation of each HA unit produces two new particles which then go their own ways, thus spreading (or "diluting") the thermal energy more extensively and massively increasing the number of energetically-equivalent microscopic states, of which entropy is a measure. (More on this here)

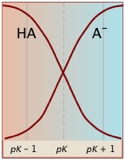
Degree of dissociation depends on the pH

Plots of this kind are discussed in more detail in the next lesson in this set under the heading ionization fractions.

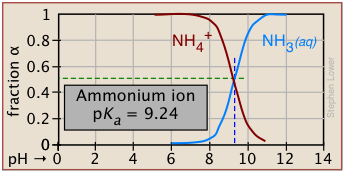The Le Châtelier principle predicts that the extent of the reaction
HA → H⁺ + A^– will be affected by the hydrogen ion concentration, and thus by the pH. This is illustrated here for the ammonium ion. Notice that when the pH is the same as the pK_a, the concentrations of the acid- and base forms of the conjugate pair are identical.

Problem Example 2 - percent dissociation

A 0.75 M solution of an acid HA has a pH of 1.6. What is its percent dissociation?

Solution: The dissociation stoichiometry HA → H⁺ + AB^– tells us the concentrations [H⁺] and [A^–] will be identical. Thus [H⁺] = 10^–1.6 = 0.025 M = [A^–]. The dissociation fraction α = [A^–] / [HA] = 0.025 / 0.75 = 0.033,
and thus the acid is 3.3% dissociated at 0.75 M concentration.

Sometimes the percent dissociation is given, and K_a must be evaluated.

Problem Example 3 - K_a from degree of dissociation

A weak acid HA is 2 percent dissociated in a 1.00 M solution. Find the value of K_a.

Solution: The equilibrium concentration of HA will be 2% smaller than its nominal concentration, so [HA] = 0.98 M, [A^–] = [H⁺] = 0.02 M. Substituting these values into the equilibrium expression gives

"Concentration of the acid" and [HA] are not the same

So for a solution made by combining 0.10 mol of pure formic acid HCOOH with sufficient water to make up a volume of 1.0 L, C_a = 0.10 M. But we know that the concentration of the actual species [HCOOH] will be smaller the 0.10 M because some it ends up as the formate ion HCOO^–. But it will always be the case that the sum [HCOOH] + [HCOO^–] = C_a.

For the general case of an acid HA, we can write a mass balance equation

C_a = [HA] + [A^–](1-16)

which reminds us the "A" part of the acid must always be somewhere!

Similarly, for a base B we can write

C_b = [B] + [HB⁺](1-17)

Degree of dissociation varies inversely with the concentration

If we represent the dissociation of a C_a M solution of a weak acid by

(1-18)

then its dissociation constant is given by

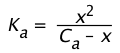(1-19)

Because the C_a term is in the denominator here, we see that the amount of HA that dissociates varies inversely with the concentration; as C_a approaches zero, [HA] approaches C_a.

If we represent the fraction of the acid that is dissociated as

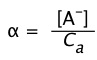(1-20)

then Eq 8 becomes

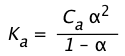(1-21)

If the acid is sufficiently weak that x does not exceed 5% of C_a,
the -term in the denominator can be dropped, yielding

K_a ≈ C_a ² (1-22)

Note that the above equations are also valid for weak bases if Kb and C_b are used in place of K_a and C_a.

Problem Example 4 - effects of dilution

Compare the percent dissociation of 0.10 M and .0010 M solutions of boric acid
(K_a = 3.8E–10).

Solution:

Boric acid is sufficiently weak that we can use the approximation of Eq 1-22 to calculate a:= (5.8E–10 / .1)^½ = 7.5E-5; multiply by 100 to get .0075 % diss. For the more dilute acid, a similar calculation yields 7.6E–4, or 0.76%.

2 Carrying out acid-base calculations

What you need to know before you start

In Problem Example 1, we calculated the pH of a monoprotic acid solution, making use of an approximation in order to avoid the need to solve a quadratic equation.

This raises the question: how "exact" must calculations of pH be?

It turns out that the relation between pH and the nominal concentration of an acid, base, or salt (and especially arbitrary mixtures of these) can become quite complicated, requiring the solution of sets of simultaneous equations.

But for almost all practical applications, and certainly those encountered in a General Chemistry course, one can make some approximations that simplify the math without detracting significantly from the accuracy of the results.

Equilibrium constants are rarely exactly known

As we pointed out in the preceding lesson, the "effective" value of an equilibrium constant (the activity) will generally be different from the value given in tables in all but the most dilute ionic solutions. Even if the acid or base itself is dilute, the presence of other "spectator" ions such as Na⁺ at concentrations much in excess of 0.001 M can introduce error.

The usual advice is to consider K_a values to be accurate to ±5 percent at best, and even more uncertain when total ionic concentrations exceed 0.1 M.

As a consequence of this uncertainty, there is generally little practical reason to express the results of a pH calculation to more than two significant digits.

Finding the pH of a solution of a weak monoprotic acid

This is by far the most common type of problem you will encounter in a first-year Chemistry class. You are given the concentration of the acid, expressed as C_a moles/L, and are asked to find the pH of the solution.

The very important first step is to make sure you understand the problem by writing down the equation expressing the concentrations of each species in terms of a single unknown, which we represent here by x:

(2-1)

Substituting these values into the expression for K_a, we obtain

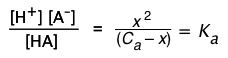(2-2)

Don't bother to memorize these equations! If you understand the concept of mass balance on "A" expressed in (2-1), and can write the expression for K_a, you just substitute the x's into the latter, and you're off! If you feel the need to memorize stuff you don't need, it is likely that you don't really understand the material — and that should be a real worry!

In order to predict the pH of this solution, we must first find [H⁺], that is, x. The presence of terms in both x and x ² here tells us that this is a quadratic equation. This can be rearranged into x ² = K_a (1 – x) which, when written in standard polynomial form, becomes the quadratic

[H⁺]² – C_a [H⁺] – K_w = 0 (2-3)

But don't panic! As we will explain farther on, in most practical cases we can make some simplifying approximations which eliminate the need to solve a quadratic. And when, as occasionally happens, a quadratic is unavoidable, we will show you some relatively painless ways of dealing with it.

How to deal with quadratic equations

What you do will depend on what tools you have available. If you are only armed with a simple calculator, then there is always the venerable quadratic formula that you may have learned about in high school, but if at all possible, you should avoid it: its direct use in the present context is somewhat laborious and susceptible to error.

Use of the standard quadratic formula on a computer or programmable calculator can lead to weird results!

The reason for this is that if b² >> |4ac|, one of the roots will require the subtraction of two terms whose values are very close; this can lead to considerable error when carried out by software that has finite precision.

One can get around this by computing the quantity

Q = –[b + sgn(b) * sqrt(b² – 4ac)]/2

from which the roots are x₁= Q /a and x₂ = c /Q. (See any textbook on numerical computing for more on this and other metnods.)

But who want's to bother with this stuff in order to solve typical chemistry problems? Better to avoid quadratics altogether if at all possible!

Remember: there are always two values of x (two roots) that satisfy a quadratic equation. For all acid-base equilibrium calculations that are properly set up, these roots will be real, and only one will be positive; this is the one you take as the answer.

Approximations, judiciously applied, simplify the math

We have already encountered two of these approximations in the examples of the preceding section:

In all but the most dilute solutions, we can assume that the dissociation of the acid HA is the sole source of H⁺, with the contribution due to water autoprotolysis being negligible.
We were able to simplify the equilibrium expressions by assuming that the x-term, representing the quantity of acid dissociated, is so small compared to the nominal concentration of the acid C_a that it can be neglected. Thus in Problem Example 1, the term in the denominator that has the form (0.1 - x) , representing the equilibrium concentration of the undissociated acid, is replaced by 0.1.

Most people working in the field of practical chemistry will never encounter situations in which the first of these approximations is invalid. This is not the case, however, for the second one.

Should I drop the x, or forge ahead with the quadratic form?

If the acid is fairly concentrated (usually with C_a > 10^–3M) and sufficiently weak that most of it remains in its protonated form HA, then the concentration of H⁺ it produces may be sufficiently small that the expression for K_a reduces to

K_a ≈ [H⁺]² / C_a

so that

[H⁺] ≈ (K_a C_a)^½(2-4)

This can be a great convenience because it avoids the need to solve a quadratic equation. But it also exposes you to the danger that this approximation may not be justified.

The usual advice is that if this first approximation of x exceeds 5 percent of the value it is being subtracted from (0.10 in the present case), then the approximation is not justified. We will call this the "five percent rule".

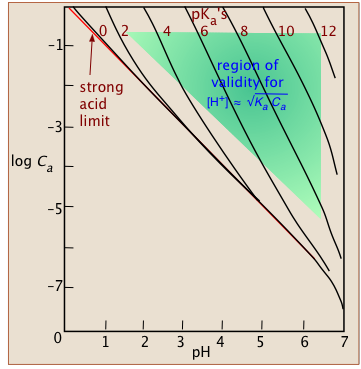
This plot shows the combinations of K_a and C_a that generally yield satisfactory results with the approximation of
Eq 4.

Problem Example 5 - pH and degree of dissociation

a) Estimate the pH of a 0.20 M solution of acetic acid, K_a = 1.8 × 10^–5.

b) What percentage of the acid is dissociated?

Substitution into the equilibrium expression yields

Can we simplify this by applying the approximation 0.20 – x ≈ 0.20 ? Looking at the number on the right side of this equation, we note that it is quite small. This means the left side must be equally small, which requires that the denominator be fairly large, so we can probably get away with dropping x. Doing so yields

(x² / 0.20) = 1.8E-5 or x = (0.20 × 1.8E–5)^½ = 1.9E-3 M

The "5 per cent rule" requires that the above result be no greater than 5% of 0.20, or 0.010. Because 0.0019 meets this condition, we can set
x = [H⁺] ≈ 1.9 × 10^–3M, and the pH will be –log (1.9 × 10^–3) = 2.7

b) Percent dissociation: 100% × (1.9 × 10^–3 M) / (0.20 M) = 0.95%

Weak bases are treated in an exactly analogous way:

Problem Example 6 - Weak base

Methylamine CH₃NH₂ is a gas whose odor is noticed around decaying fish. A solution of CH₃NH₂ in water acts as a weak base. A 0.10 M solution of this amine in water is found to be 6.4% ionized. Use this information to find \Kb and pK_b for methylamine.

Solution: When methylamine "ionizes", it takes up a proton from water, forming the methylaminium ion:

CH₃NH₂ + H₂O → CH₃NH₃⁺ + OH^–

Let x = [CH₃NH₃⁺] = [OH^–] = .064 × 0.10 = 0.0064

[CH₃NH₂] = (0.10 – .064) = 0.094

Substitute these values into equilibrium expression for \Kb:

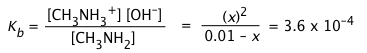
To make sure we can stop here, we note that (3.6E4 / .01) = .036; this is smaller than .05, so we pass the 5% rule and can use the approximation and drop the
x-term in the denominator.

pK_b = – log \Kb = – log (4.4 × 10^–10) = 3.36

But one does not always get off so easily!

Problem Example 7 - pH of a chloric acid solution

With a K_a of 0.010, HClO₂ is one of the "stronger" weak acids, thanks to the two oxygen atoms whose electronegativity withdraws some negative charge from the chlorine atom, making it easier for the hydrogen to depart as a proton.

Find the pH of a 0.015 M solution of chloric acid in pure water.

(i)

The approximation 0.10 – x ≈ .015 gives us

x ≈ (K_a C_a)^½ = (0.10 ×.010)^½ = (.001)^½ = .032 (ii)

This result should should sound alarm bells in your head right away; here is no way that one can get 0.032 mole of H⁺ from 0.010 mole of even the strongest acid!

The difficulty, in this case, arises from the numerical value of K_a differing from the nominal concentration 0.10 M by only a factor of 10. As a result,
x / C_a = .032 / 0.10 = 0.32 which clearly exceeds the 5% limit; we have no choice but to face the full monte of the quadratic solution. (see Problem Example 8 below).

Successive approximations will get you there with minimal math

In the method of successive approximations, you start with the value of [H⁺] (that is, x) you calculated according to (2-4), which becomes the first approximation. You then substitute this into (2-2), which you solve to get a second approximation. This cycle is repeated until differences between successive answers become small enough to ignore.

Problem Example 8 - Method of successive approximations

Estimate the pH of a 0.10 M aqueous solution of HClO₂, K_a = 0.010, using the method of successive approximations.

Solution: The equilibrium condition is

(i)

We solve this for x, resulting in the first approximation x₁, and then successively plug each result into the previous equation, yielding approximations x₂ and x₃:

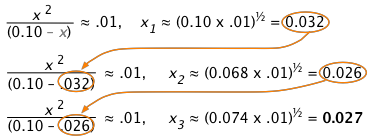(ii)

The last two approximations x₂ and x₃ are within 5% of each other.
Note that if we had used x₁as the answer, the error would have been 18%.

(An exact numeric solution yields the roots 0.027016 and –0.037016)

Use a graphic calculator or computer to find the positive root

The real roots of a polynomial equation can be found simply by plotting its value as a function of one of the variables it contains.

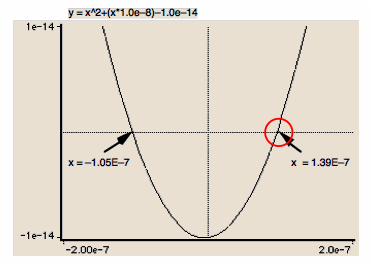
In this example, the pH of a 10^–6 M solution of hypochlorous acid (HOCl, K_a = 2.9E–8) was found by plotting the value of
y = ax² + bx + c, whose roots are the two values of x that correspond to y = 0.

This method generally requires a bit of informed trial-and-error to make the locations of the roots visible within the scale of the axes.

Be lazy, and use an on-line quadratic equation solver

If you google "quadratic equation solver", you will find numerous on-line sites that offer quick-and-easy "fill-in-the-blanks" solutions.

Unfortunately, few of these will be useful for acid-base problems involving numbers that must be expressed in "E-notation" (e.g., 2.7E-11.) Of those that do, the one at the MathIsFun site is highly recommended; others can be found here and at the Quad2Deg site.

If you can access a quad equation solver on your personal electronic device or through the Internet, this is quick and painless. All you need to do is write the equation in polynomial form ax² + bx + c = 0, insert values for
a, b, and c, and away you go!

This is so easy, that many people prefer to avoid the "5% test" altogether, and go straight to an exact solution. But make sure can do the 5%-thing for exams where Internet-accessible devices are not permitted!

Problem Example 9 - chloric acid, again

Estimate the pH of a 0.10 M aqueous solution of HClO₂, K_a = 0.010.

Solution: The reaction equation HClO₂ → H⁺ + ClO₂– defines the equilibrium expression

Multiplying the right half of the above expression yields

x² = 0.010 × (0.10 – x) = .0010 – .01 x which we arrange into standard polynomial form:

x² + 0.01 x – 0.0010 = 0

Entering the coefficients {1 .01 –.001} into an online quad solver yields the roots
.027 and –.037. Taking the positive one, we have [H⁺] = .027 M;
the solution pH is – log .027 = 1.6.

Note: a common error is to forget to enter the minus sign for the last term; try doing this and watch the program blow up!

Avoid math altogether and make a log-C vs pH plot

This is not only simple to do (all you need is a scrap of paper and a straightedge), but it will give you far more insight into what's going on, especially in polyprotic systems. All explained in Section 3 of the next lesson.

Some videos on simple monoprotic acid-base calculations:

pH of a weak acid (Khan, 18 min)
pH of a weak acid (ChemGuy,6.½ min)
pH of a weak base (Khan, 13 min)
pH of a weak base carbonate and bicarbonate ions (ChemGuy, 6.½ min)

Solutions of salts

Most salts do not form pH-neutral solutions

Salts such as sodium chloride that can be made by combining a strong acid (HCl) with a strong base (NaOH, KOH) have a neutral pH, but these are exceptions to the general rule that solutions of most salts are mildly acidic or alkaline.

Salts of a strong base and a weak acid yield alkaline solutions.

"Hydro-lysis" literally means "water splitting", as exemplified by the reaction A^– + H₂O → HA + OH^–. The term describes what was believed to happen prior to the development of the Brønsted-Lowry proton transfer model.

This important property has historically been known as hydrolysis — a term still used by chemists.

Some examples:

Potassium Cyanide: KCN can be thought of the salt made by combining the strong base KOH with the weak acid HCN: K⁺ + OH^– → KOH. When solid KCN dissolves in water, this process is reversed, yielding a solution of the two ions. K⁺, being a "strong ion", does not react with water. But the "weak ion" CN^–, being the conjugate base of a weak acid, will have a tendency to abstract protons from water: CN^– + H₂O → HCN + OH^–, causing the solution to remain slightly alkaline.
Sodium bicarbonate: NaHCO₃ (more properly known as sodium hydrogen carbonate) dissolves in water to yield a solution of the hydrogen carbonate ion HCO₃^–. This ion is an ampholyte — that is, it is both the conjugate base of the weak carbonic acid H₂CO₃, as well as the conjugate acid of the carbonate ion CO₃^2–:

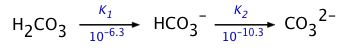
The HCO₃^– ion is therefore amphiprotic: it can both accept and donate protons, so both processes take place:

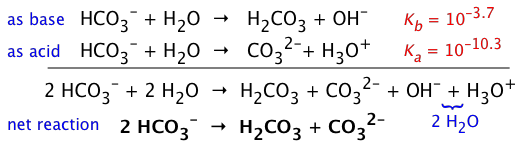
But if we compare the K_a and K_b of HCO₃^–, it is apparent that its basic nature wins out, so a solution of NaHCO₃ will be slightly alkaline. (The value of pK_b is found by recalling that K_a + K_b = 14.)

Sodium acetate: A solution of CH₃COONa serves as a model for the strong-ion salt of any organic acid, since all such acids are weak: CH₃COO^– + H₂O → CH₃COOH + OH^–

Salts of most cations (positive ions) give acidic solutions

The protons can either come from the cation itself (as with the ammonium ion NH₄⁺), or from waters of hydration that are attached to a metallic ion.

This latter effect happens with virtually all salts of metals beyond Group I; it is especially noticeable for salts of transition ions such as hexaaquoiron(III) ("ferric ion"):

Fe(H₂O)₆³⁺ → Fe(H₂O)₅OH²⁺ + H⁺ (2-5)

This comes about because the positive field of the metal enhances the ability of H₂O molecules in the hydration shell to lose protons. In the case of the hexahyrated ion shown above, a whose succession of similar steps can occur, but for most practical purposes only the first step is significant.

Problem Example 10 - Aluminum chloride solution

Find the pH of a 0.15 M solution of aluminum chloride.

Solution: The aluminum ion exists in water as hexaaquoaluminum Al(H₂O)₆³⁺, whose pK_a = 4.9, K_a = 10^–4.9 = 1.3E–5. Setting x = [H⁺] = [Al(H₂O)₅OH ²⁺], the equilibrium expression is

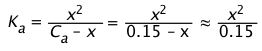
Using the above approximation, we get
x ≈ (1.96E–6)^½ = 1.4E–3, corresponding to pH = 2.8.

Finally, we compute x/C_a = 1.4E–3 ÷ 0.15 = .012 confirming that we are within the "5% rule".

The only commonly-encountered salts in which the proton is donated by the cation itself are those of the ammonium ion:

NH₄+→ NH₃(aq) + H⁺(2-6)

Problem Example 11 - Ammonium chloride solution

Calculate the pH of a 0.15 M solution of NH4Cl. The ammonium ion K_a is 5.5E–10.

Solution: According to Eq 6 above, we can set [NH3] = [H⁺] = x, obtaining the same equilibrium expression as in the preceding problem.

Because K_a is quite small, we can safely use the approximation 0.15 - 1 ≈ .015, which yields pH = –log 0.90E–5 = 5.0

Most salts of weak acids form alkaline solutions

As indicated in the example, such equilibria strongly favor the left side; the stronger the acid HA, the less alkaline the salt solution will be.

Because an ion derived from a weak acid such as HF is the conjugate base of that acid, it should not surprise you that a salt such as NaF forms an alkaline solution, even if the equilibrium greatly favors the left side::

F^– + H₂O HF + OH^–(2-7)

Problem Example 11 - Solution of sodium fluoride

Find the pH of a 0.15 M solution of NaF. (HF K_a = 6.7E–4)

Solution: The reaction is F- + H₂O = HF + OH^–; because HF is a weak acid, the equilibrium strongly favors the right side. The usual approximation yields

But on calculating x/C_a = .01 ÷ 0.15 = .07, we find that this does not meet the "5% rule" for the validity of the approximation. We therefore expand the equilibrium expression

into standard polynomial form x² + 6.7E–4 x – 1.0E–4 = 0 and enter the coefficients {1 6.7E–4 –.0001} into a quadratic solver. This yields the positive root x = 0.0099 which turns out to be sufficiently close to the approximation that we could have retained it after all.. perhaps 5% is a bit too restrictive for 2-significant digit calculations!

What about a salt of a weak acid and a weak base?

A salt of a weak acid gives an alkaline solution, while that of a weak base yields an acidic solution. What happens if we dissolve a salt of a weak acid and a weak base in water? Ah, this can get a bit tricky! Nevertheless, this situation arises very frequently in applications as diverse as physiological chemistry and geochemistry.

As an example of how one might approach such a problem, consider a solution of ammonium formate, which contains the ions NH₄⁺ and HCOO-. Formic acid, the simplest organic acid, has a pK_a of 3.7; for NH₄⁺, pK_a = 9.3.

Three equilibria involving these ions are possible here; in addition to the reactions of the ammonium and formate ions with water, we must also take into account their tendency to react with each other to form the parent neutral species:

NH₄⁺ → NH₃ + H⁺	K₁ = 10^–9.3
HCOO^– + H₂O → HCOOH + OH^–	K₂ = (1O^–14/10^–3.7) = 10^–10.3
NH₄⁺ + HCOO^– → NH₃ + HCOOH	K₃

Inspection reveals that the last equation above is the sum of the first two,plus the reverse of the dissociation of water

H⁺ + OH^– → H₂O

K₄ = 1/K_w

The value of K₃ is therefore

(2-8)

A rigorous treatment of this system would require that we solve these equations simultaneously with the charge balance and the two mass balance equations. However, because K₃ is several orders of magnitude greater than K₁ or K₂, we can greatly simplify things by neglecting the other equilibria and considering only the reaction between the ammonium and formate ions.

Notice that the products of this reaction will tend to suppress the extent of the first two equilibria, reducing their importance even more than the relative values of the equilibrium constants would indicate.

Problem Example 12 - ammonium formate

Estimate the pH of a 0.0100 M solution of ammonium formate in water.

Solution: From the stoichiometry of HCOONH₄,

[NH₄⁺] = [HCOO^–] and [NH₃] = [HCOOH] (i)

then, from Eq 8 above,

(ii)

in which K_b is the base constant of ammonia, K_w /10^–9.3.

From the formic acid dissociation equilibrium we have

(iii)

We now rewrite the expression for K₃

(iv)

which yields

(v)

and thus the pH is 6.5

What is interesting about this last example is that the pH of the solution is apparently independent of the concentration of the salt. If K_a = K_b, then this is always true and the solution will be neutral (neglecting activity effects in solutions of high ionic strength). Otherwise, it is only an approximation that remains valid as long as the salt concentration is substantially larger than the magnitude of either equilibrium constant. Clearly, the pH of any solution must approach that of pure water as the solution becomes more dilute.

Salts of analyte ions

Polyprotic acids form multiple anions; those that can themselves donate protons, and are thus amphiprotic, are called analytes. The most widely known of these is the bicarbonate (hydrogen carbonate) ion, HCO₃^–, which we commonly know in the form of its sodium salt NaHCO₃ as baking soda.

The other analyte series that is widely encountered, especially in biochemistry, is those derived from phosphoric acid:

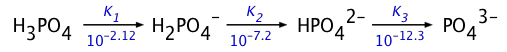
The solutions of analyte ions we most often need to deal with are the of "strong ions", usually Na⁺, but sometimes those of Group 2 cations such as Ca²⁺.

The exact treatment of these systems is generally rather complicated, but for the special cases in which the successive K_a's of the parent acid are separated by several orders of magnitude (as in the two systems illustrated above), a series of approximations reduces the problem to the simple expression

(2-9)

which, you will notice, as with the salt of a weak acid and a weak base discussed in the preceding subsection predicts that the pH is independent of the salt's concentration. This, of course, is a sure indication that this treatment is incomplete. Fortunately, however, it works reasonably well for most practical purposes, which commonly involve buffer solutions.

Exact treatment of solutions of weak acids and bases

When dealing with acid-base systems having very small K_a's, and/or solutions that are extremely dilute, it may be necessary to consider all the species present in the solution, including minor ones such as OH^–.

This is almost never required in first-year courses. But for students in more advanced courses, this "comprehensive approach" (as it is often called) illustrates the important general methodology of dealing with more complex equilibrium problems. It also shows explicitly how making various approximations gradually simplifies the treatment of more complex systems.

In order to keep the size of the present lesson within reasonable bounds (and to shield the sensitive eyes of beginners from the shock of confronting simultaneous equations), this material has been placed in a separate lesson.

3 Solutions of polyprotic acids

A diprotic acid H₂A can donate its protons in two steps:

H₂A → HA^– →HA^–

and similarly, for a tripotic acid H₃A:

H₃A → H₂A^– → HA^2– → A^3–

In general, we can expect K_a2 for the "second ionization" to be smaller than K_a1 for the first step because it is more difficult to remove a proton from a negatively charged species. The magnitude of this difference depends very much on whether the two removable protons are linked to the same atom, or to separate atoms spaced farther apart.

Some polyprotic acids you should know

These acids are listed in the order of decreasing K_a1. The numbers above the arrows show the successive K_a's of each acid.

Notice how successive K_a's of each acid become smaller, and how their ratios relate to the structures of each acid.
Sulfuric acid is the only strong polyprotic acid you are likely to encounter.
Sulfurous acid has never been detected; what we refer to as H₂SO₃ is more properly represented as a hydrated form of sulfur dioxide: which dissociates into the hydrosulfite ion:
SO₂·H₂O → HSO₃^– + H⁺ . The ion HSO₃^– exists only in solution; solid bisulfite salts are not known.
Similarly, pure carbonic acid has never been isolated, but it does exist as a minority species in an aqueous solution of CO₂: [CO₂(aq)] = 650[H₂CO₃]. The formula H₂CO₃ ordinarily represents the combination CO₂(aq) and "true" H₂CO₃. The latter is actually about a thousand times stronger than is indicated by the pK_a of 6.3, which is the weighted average of the equilibrium mixture.

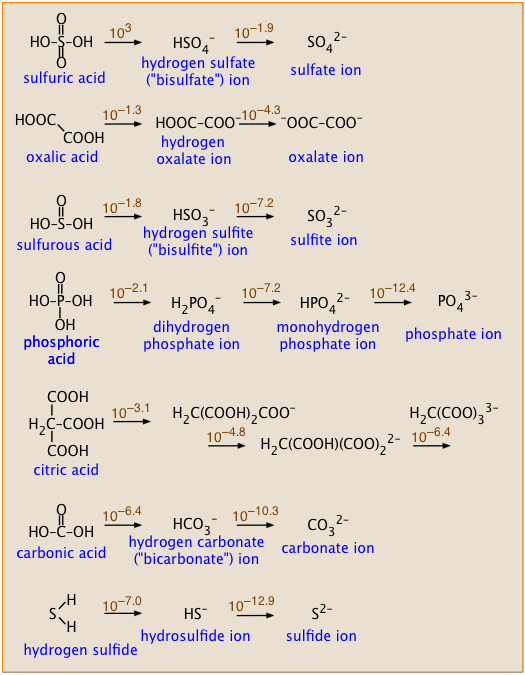
common polyprotic acids

pH of a polyprotic acid (LindaHanson, 17 min)

Problem Example 13 - Comparison of two diprotic acids

Compare the successive pK_a values of sulfuric and oxalic acids (see their structures in the box, above right), and explain why they should be so different.

Solution: The two pK_a values of sulfuric acid differ by 3.0 – (–1.9) = 4.9, whereas for oxalic acid the difference is 1.3 – (–4.3) = 3.0. That's a difference of almost 100 between the two K_a's. Removal of a second proton from a molecule that already carries some negative charge is always expected to be less favorable energetically. In sulfuric acid, the two protons come from –OH groups connected to the same sulfur atom, so the negative charge that impedes loss of the second proton is more localized near the site of its removal. In oxalic acid, the two protons are removed from –OH groups attached to separate carbon atoms, so the negative charge of the mono-negative ions will exert less restraint on loss of the second proton.

Solutions of polyprotic acids in pure water

With the exception of sulfuric acid (and some other seldom-encountered strong diprotic acids), most polyprotic acids have sufficiently small K_a1 values that their aqueous solutions contain significant concentrations of the free acid as well as of the various dissociated anions.

Thus for a typical diprotic acid H₂A, we must consider the equilibria

H₂A → H⁺ + HA^– K₁

HA^–→ H⁺ + HA^2– K₂

H₂O → H⁺ + OH^– K_w

An exact treatment of such a system of four unknowns [H₂A], [HA^–], [A^2–] and [H⁺] requires the solution of a quartic equation. If we include [OH^–], it's even worse!

In most practical cases, we can make some simplifying approximations:

Unless the solution is extremely dilute or K₁ (and all the subsequent K's) are extremely small, we can forget that any hydroxide ions are present at all.
If K₁ is quite small, and the ratios of succeeding K's are reasonably large, we may be able, without introducing too much error, to neglect the other K's and treat the acid as monoprotic.

In addition to the three equilibria listed above, a solution of a polyprotic acid in pure water is subject to the following two conditions:

Material balance: although the distribution of species between the acid form H₂A and its base forms HAB^– and A^2–may vary, their sum (defined as the "total acid concentration" C_a is a constant for a particular solution:

C_a = [H₂A] + [HA^–] + [A^2–]

Charge balance: The solution may not possess a net electrical charge:

[H₃O⁺] = [OH^–] + [HA^–] + 2 [A^2–]

Why do we multiply [A^2–] by 2? Two moles of H₃O⁺ are needed in order to balance out the charge of 1 mole of A^2–.

Simplified treatment of polyprotic acid solutions

The calculations shown in this section are all you need for the polyprotic acid problems encountered in most first-year college chemistry courses. More advanced courses may require the more exact methods in Lesson 7.

Because the successive equilibrium constants for most of the weak polyprotic acids we ordinarily deal with diminish by several orders of magnitude, we can usually get away with considering only the first ionization step. To the extent that this is true, there is nothing really new to learn here. However, without getting into a lot of complicated arithmetic, we can often go farther and estimate the additional quantity of H⁺ produced by the second ionization step.

Problem Example 14 - Solution of CO₂ in water

a) Calculate the pH of a 0.050 M solution of CO₂ in water.
For H₂CO₃, K₁ = 10^–6.4 = 4.5E–7, K₂ = 10^–10.3 = 1.0E–14.

b) Estimate the concentration of carbonate ion CO₃^2– in the solution.

Solution:

a) Because K₁ and K₂ differ by almost four orders of magnitude, we will initially neglect the second dissociation step. Because this latter step produces only a tiny additional concentration of H⁺, we can assume that [H⁺] = [HCO₃^–] = x:

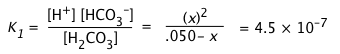(i)

Can we further simplify this expression by dropping the x in the denominator? Let's try: x = [0.05 × (4.5E–7)]^½ = 1.5E–4.

Applying the "5-percent test", the quotient x/C_a must not exceed 0.05. Working this out yields (1.5E–4)/(.05) = .003, so we can avoid a quadratic.

x = [H⁺] ≈ (K_aC_a)½ = [(4.5E–7) × .01]^½ = (.001)½ = 0.032 M,
and the pH = – log .032 = 1.5.

b) We now wish to estimate [CO₃^2–] ≡ x.

Examining the second dissociation step, it is evident that this will consume x mol/L of HCO₃^–, and produce an equivalent amount of H⁺ which adds to the quantity we calculated in (a).

(ii)

Owing to the very small value of K₂ compared to K₁, we can assume that the concentrations of HCO₃^– and H⁺ produced in the first dissociation step will not be significantly altered in this step. This allows us to simplify the equilibrium constant expression and solve directly for [CO₃^2–]:

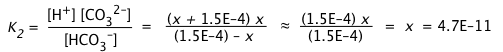(iii)

It is of course no coincidence that this estimate of [CO₃^2–] yields a value identical with K₂; this is entirely a consequence of the simplifying assumptions we have made. Nevertheless, as long as K₂ << K₁ and the solution is not highly dilute, the result will be sufficiently accurate for most purposes.

Sulfuric acid: a special case

Although this is a strong acid, it is also diprotic, and in its second dissociation step it acts as a weak acid. Because sulfuric acid is so widely employed both in the laboratory and industry, it is useful to examine the result of taking its second dissociation into consideration.

Problem Example 15 - solution of H₂SO₄

Estimate the pH of a 0.010 M solution of H₂SO₄. K₁ = 10³, K₂ = 0.012

Solution: Because K₁ > 1, we can assume that a solution of this acid will be completely dissociated into H₃O⁺ and bisulfite ions HSO₄^–. Thus the only equilibrium we need to consider is the dissociation of a 0.010 M solution of bisulfite ions.

HSO₄^– + H₂O → SO₄^2– + H₃O⁺

Setting [H⁺] = [SO₄^2–] = x, and dropping x from the denominator, yields
x ≈ (0.010 x .012)^½ = (1.2E–4)^½ = 0.0011

Applying the "five percent rule", we find that x / C_a = .0011/.01 = .11 which is far over the allowable error, so we must proceed with the quadratic form. Rewriting the equilibrium expression in polynomial form gives

x² + 0.022x – 1.2E–4 = 0

Inserting the coefficients {1 .022 .000012} into a quad-solver utility yields the roots 4.5E–3 and –0.0027. Taking the positive root, we obtain

pH = – log (.0045) = 2.3

Thus the second "ionization" of H₂SO₄ has only reduced the pH of the solution by 0.1 unit from the value (2.0) it would theoretically have if only the first step were considered.

4 Amino acids and Zwitterions

Most first-year General Chemistry students may skip this section.
This material is covered mainly in biochemistry courses.

Zwitterions: molecular hermaphrodites

Amino acids, the building blocks of proteins, contain amino groups –NH₂ that can accept protons, and carboxyl groups –COOH that can lose protons. Under certain conditions, these events can occur simultaneously, so that the resulting molecule becomes a “double ion” which goes by its German name Zwitterion.

Glycine: the simplest amino acid

The simplest of the twenty natural amino acids that occur in proteins is glycine H₂N–CH₂–COOH, which we use as an example here. Solutions of glycine are distributed between the acidic-, zwitterion-, and basic species:

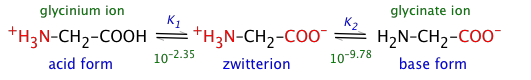(3-1)

Although the zwitterionic species is amphiprotic, it differs from a typical ampholyte such as HCO₃^– in that it is electrically neutral owing to the cancellation of the opposite electrical charges on the amino and carboxyl groups.

Amino acids are the most commonly-encountered kind of zwitterions, but other substances, such as quaternary ammonium compounds, also fall into this category. For more on Zwitterions, see this Wikipedia article or this UK ChemGuide page.

In the following development, we use the abbreviations H₂Gly⁺ (glycinium), HGly (zwitterion), and Gly^– (glycinate) to denote the dissolved forms.

The two acidity constants are

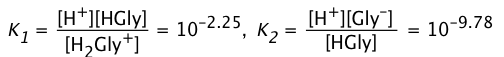(3-2)

If glycine is dissolved in water, charge balance requires that

H₂Gly⁺ + [H⁺] = [Gly^–] + [OH^–](3-3)

Substituting the equilibrium constant expressions (including that for the autoprotolysis of water) into the above relation yields

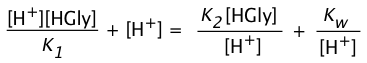(3-4)

These videos discuss the pH-related chemistry of amino acids:

Amino acid pK_a’s and their acid/base forms (UCBerkeley, 7 min)
Net charge and isoelectric point of an amino acid (Khan, 5½ min)
Amino acid effective charge - quiz (UCBerkeley, 3 min)

If Eqs ii and iii in this Problem Example are recalculated for a range of pH values, one can plot the concentrations of each species against pH for 0.10 M glycine in water:

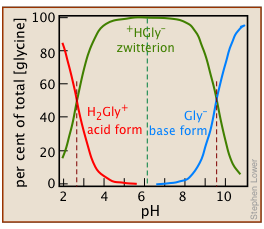
This distribution diagram shows that the zwitterion is the predominant species between pH values corresponding to the two pK_as given in Eq 3-1.

Problem Example 16 - glycine solution speciation

Calculate the pH and the concentrations of the various species in a 0.100 M solution of glycine.

Solution: Substitution into Eq 4 above yields

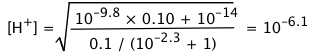(i)

The concentrations of the acid and base forms are found from their respective equilibrium constant expressions (Eqs 2):

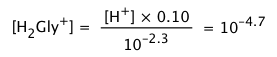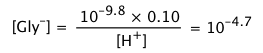(ii)

(iii)

The small concentrations of these singly-charged species in relation to C_a = 0.10 shows that the zwitterion is the only significant glycine species in the solution.

What you should be able to do

Make sure you thoroughly understand the following essential concepts that have been presented above.

Explain the difference between the terms C_a and [HA] as they relate to an aqueous solution of the acid HA.
Define degree of dissociation and sketch a plot showing how the values of 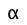for a conjugate pair HA and A^– relate to each other and to the pK_a.
Derive Eq 2-2 relating the acid concentration and dissociation constant to the hydrogen ion concentration in a solution of a weak acid.
Re-write the above relation in polynomial form.
Derive the approximation (Eq 2-4) that is often used to estimate the pH of a solution of a weak acid in water.
Calculate the pH of a solution of a weak monoprotic weak acid or base, employing the "five-percent rule" to determine if the approximation 2-4 is justified.
Predict whether an aqueous solution of a salt will be acidic or alkaline, and explain why by writing an appropriate equation.