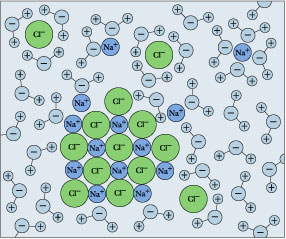
NaCl(s) → Na+(aq) + Cl–(aq)
[Image from U. of Virginia]
The designation (aq) means "aqueous" and comes from aqua, the Latin word for water. It is used to indicate that the ions are dissolved in water, and to remind us that these ions are hydrated — meaning that H2O molecules are attached to them.
Remember that solubility equilibrium and the calculations that relate to it are only meaningful when both sides (solids and dissolved ions) are simultaneously present.
But if you keep adding salt, there will come a point at which it no longer seems to dissolve. If this condition persists, we say that the salt has reached its solubility limit, and the solution is saturated in NaCl. The situation is now described by
NaCl(s) ![]() Na+(aq) + Cl–(aq)
Na+(aq) + Cl–(aq)
in which the solid and its ions are in equilibrium.
Salt solutions that have reached or exceeded their solubility limits (usually 36-39 g per 100 mL of water) are responsible for prominent features of the earth's geochemistry. They typically form when NaCl leaches from soils into waters that flow into salt lakes in arid regions that have no natural outlets; subsequent evaporation of these brines force the above equilibrium to the left, forming natural salt deposits. These are often admixed with other salts, but in some cases are almost pure NaCl.
Many parts of the world contain buried deposits of NaCl (known as halite) that formed from the evaporation of ancient seas, and which are now mined.
Below: some interesting salt geochemistry [click on thumbnails to enlarge them]
 glaciers (dark gray) protruding through in Iran [NASA]
glaciers (dark gray) protruding through in Iran [NASA] 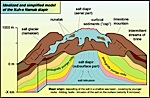 Structure of a salt diapir
Structure of a salt diapir
 Evaporative salt pans in San Francisco Bay (colors due to bacteria and algae)
Evaporative salt pans in San Francisco Bay (colors due to bacteria and algae)  Wieliczka Salt Mine near Krakow, Poland
Wieliczka Salt Mine near Krakow, Poland
How solubilities are expressed
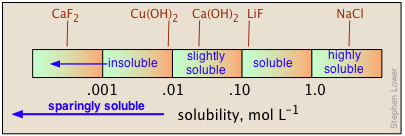
Solubilities are most fundamentally expressed in molar (mol L–1 of solution) or molal (mol kg–1 of water) units. But for practical use in preparing stock solutions, chemistry handbooks usually express solubilities in terms of grams-per-100 ml of water at a given temperature, frequently noting the latter in a superscript. Thus 6.9 20 means 6.9 g of solute will dissolve in 100 mL of water at 20° C.
When quantitative data are lacking, the designations "soluble", "insoluble", "slightly soluble", and "highly soluble" are used. There is no agreed-on standard for these classifications, but a useful guideline might be that shown below.
What factors determine the solubility of a salt in water?
The solubilities of salts in water span a remarkably large range of values, from almost completely insoluble to highly soluble. Moreover, there is no simple way of predicting these values, or even of explaining the trends that are observed for the solubilities of different anions within a given group of the periodic table.
A more extended discussion of ionic solid dissolution energetics is given in another lesson in this series.
Ultimately, the driving force for dissolution (and for all chemical processes) is determined by the Gibbs free energy change. But because many courses cover solubility before introducing free energy, we will not pursue this here.
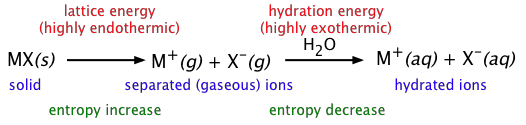
Dissolution of a salt is conceptually understood as a sequence of the two processes depicted above: breakup of the ionic lattice of the solid, followed by attachment of water molecules to the released ions. The first step consumes a large quantity of energy, something that by itself would strongly discourage solubility. But the second step releases a large amount of energy and thus has the opposite effect.
Thus the net energy change depends on the sum of two large energy terms (often approaching 1000 kJ/mol) having opposite signs. Each of these terms will to some extent be influenced by the size, charge, and polarizability of the particular ions involved, and on the lattice structure of the solid. This large number of variables makes it impossible to predict the solubility of a given salt.
Nevertheless, there are some clear trends for how the solubilities of a series of salts of a given anion (such as hydroxides, sulfates, etc.) change with a periodic table group. And of course, there are a number of general solubility rules — for example, that all nitrates are soluble, while most sulfides are insoluble.
Solubility and temperature
Solubility usually increases with temperature - but not always
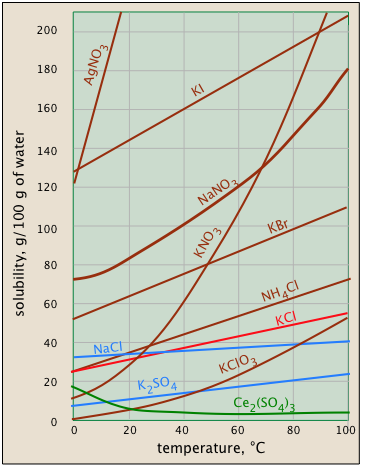
The temperature dependence of any process depends on its entropy change — that is, on the degree to which thermal kinetic energy can spread throughout the system. When a solid dissolves, its component molecules or ions diffuse into the much greater volume of the solution, carrying their thermal energy along with them. So we would normally expect the entropy to increase — something that makes any process take place to a greater extent at a higher temperature.
So why does the solubility of cerium sulfate (green plot) diminish with temperature? Dispersal of the Ce3+ and SO42– ions themselves is still associated with an entropy increase, but in this case the entropy of the water decreases even more owing to the ordering of the H2O molecules that attach to the Ce3+ ions as they become hydrated. It's difficult to predict these effects, or explain why they occur in individual cases — but they do happen.
Sparingly soluble solids: why they are important
Sparingly soluble solids are commonly said to be "insoluble".
All solids that dissociate into ions exhibit some limit to their solubilities, but those whose saturated solutions exceed about 0.01 mol L–1 cannot be treated by simple equilibrium constants owing to ion-pair formation that greatly complicates their behavior. For this reason, most of what follows in this lesson is limited to salts that fall into the "sparingly soluble" category.
"Insoluble" salts drive double-displacement (metathesis) reactions to completion
The importance of sparingly soluble solids arises from the fact that formation of such a product can effectively remove the corresponding ions from the solution, thus driving the reaction to the right.
Consider, for example, what happens when we mix solutions of strontium nitrate and potassium chloride in a 1:2 mole ratio. Although we might represent this process by
Sr(NO3)2(aq) + 2 KCl(aq) → SrCl(aq) + 2 KNO3(aq)(1)
the net ionic equation
Sr2+ + 2 NO3– + 2 K+ + 2 Cl– → Sr2+ + 2 NO3– + 2 K+ + 2 Cl–
indicates that no net change at all has taken place!
Of course if the solution were than evaporated to dryness, we would end up with a mixture of the four salts shown in Eq. 1, so in this case we might say that the reaction is half-complete.
Contrast this with what happens if we combine equimolar solutions of barium chloride and sodium sulfate:
BaCl2(aq) + Na2SO4(aq) → 2 NaCl(aq) + BaSO4(s)(2)
whose net ionic equation is
Ba2+ + 2 Cl– + 2 Na+ + SO42– → 2 Na+ + 2 Cl– + BaSO4(s)
which after canceling out like terms on both sides, becomes simply
Ba2+ + SO42– → BaSO4(s)(3)
Insoluble salts form the basis of "wet chemistry" analysis
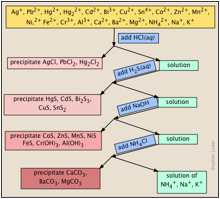
The standard qualitative analysis separation scheme
Because the formation of sparingly soluble solids is "complete" (that is, equilibria such as the one shown above for barium sulfate lie so far to the right), virtually all of one or both of the contributing ions are essentially removed from the solution. Such reactions are said to be quantitative, and they are especially important in analytical chemistry:
- Qualitative analysis
- This most commonly refers to a procedural scheme, widely encountered in first-year laboratory courses, in which a mixture of cations (usually in the form of their dissolved nitrate salts) is systematically separated and identified on the basis of the solubilities of their various anion salts such as chlorides, carbonates, sulfates, and sulfides.
- Although this form of qualitative analysis is no longer employed by modern-day chemists (instrumental techniques such as atomic absorption spectroscopy are much faster and comprehensive), it is still valued as an educational tool for familiarizing students with some of the major classes of inorganic salts, and for developing basic skills relating to observing, organizing, and interpreting results in the laboratory.
- Quantitative gravimetric analysis
- In this classical form of chemical analysis, an insoluble salt of a cation is prepared by precipitating it by addition of a suitable anion. The precipitate is then collected, dried, and weighed ("gravimetry") in order to determine the concentration of the cation in the sample.
- For example, a gravimetric procedure for determining the quantity of barium in a sample might involve precipitating the metal as the sulfate according to Eq. 3 above, using an excess of sulfate ion to ensure complete removal of the barium.
- This method of quantitative analysis became extremely important in the latter half of the nineteenth century, by which time reasonably accurate atomic weights had become available, and sensitive analytical balances had been developed. It was not until the 1960's that it became largely supplanted by instrumental techniques which were much quicker and accurate.
- Gravimetric analysis is still usually included as a part of more advanced laboratory instruction, largely as a means of developing careful laboratory technique.
Using silver chromate as an example, we express its dissolution in water as
Ag2CrO4(s) → 2 Ag+(aq) + CrO42–(aq)(4a)
When this process reaches equilibrium (which requires that some solid be present), we can write (leaving out the "(aq)s" for simplicity)
Ag2CrO4(s) ![]() 2 Ag+ + CrO42– (4b)
2 Ag+ + CrO42– (4b)
The equilibrium constant is formally
K = [Ag+]2 [CrO42–] / [Ag2CrO4(s)](5a)
But because solid substances do not normally appear in equilibrium expressions, the equilibrium constant for this process is
[Ag+]2 [CrO42–] = Ks = 2.76E–12(5b)
Because equilibrium constants of this kind are written as products, the resulting K's are commonly known as solubility products, denoted by Ks or sometimes Ksp.
Strictly speaking, concentration units do not appear in equilibrium constant expressions. But many instructors prefer that students show them anyway, especially when using solubility products to calculate concentrations. If this is done, Ks in Eq. 5b would have units of mol3 L–3.
Saturated and unsaturated solutions
Don't confuse ion products with the solubility product
The ion product of a salt can have any value up to its limiting value which is known as its solubility product.
An expression such as [Ag+]2 [CrO42–] in known generally as an ion product — this one being the ion product for silver chromate. An ion product can in principle have any positive value, depending on the concentrations of the ions involved. Only in the special case when its value is identical with Ks does it become the solubility product. A solution in which this is the case is said to be saturated. Thus when [Ag+]2 [CrO42–] = 2.76E-12 at the temperature and pressure at which this value Ks of applies, we say that the "solution is saturated in silver chromate".
A solution must be saturated to be in equilibrium with the solid. This is a necessary condition for solubility equilibrium, but it is not by itself sufficient. True chemical equilibrium can only occur when all components are simultaneously present.
A solubility system can be in equilibrium only when some of the solid is in contact with a saturated solution of its ions.
Failure to appreciate this is a very common cause of errors in solving solubility problems.
Undersaturated and supersaturated solutions
If the ion product is smaller than the solubility product, the system is not in equilibrium and no solid can be present. Such a solution is said to be undersaturated.
A supersaturated solution is one in which the ion product exceeds the solubility product. A supersaturated solution is not at equilibrium, and no solid can ordinarily be present in such a solution. If some of the solid is added, the excess ions precipitate out and until solubility equilibrium is achieved.
How to know the saturation status of a solution
This is just a simple matter of comparing the ion product Qs with the solubility product Ks. Recall that
This table is taken from the Chemical Equilibrium lesson Q and K: What's the difference? — which you might wish to review.
| Q/K | |
| > 1 | Product concentration too high for equilibrium; net reaction proceeds to left. |
| = 1 | System is at equilibrium; no net change will occur. |
| < 1 | Product concentration too low for equilibrium; net reaction proceeds to right. |
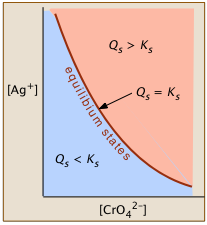 so for the system
so for the system
Ag2CrO4(s)  2 Ag+ + CrO42– (4b)
2 Ag+ + CrO42– (4b)
a solution in which Qs < Ks (i.e., Ks /Qs > 1) is undersaturated (blue shading) and the no solid will be present. The combinations of [Ag+] and [CrO42–] that correspond to a saturated solution (and thus to equilibrium) are limited to those described by the curved line. The pink area to the right of this curve represents a supersaturated solution.
How are solubilities measured?
There are two principal methods, neither of which is all that reliable for sparingly soluble salts:
- Evaporate a saturated solution of the solid to dryness, and weigh what's left.
- Measure the electrical conductivity of the saturated solution, which will be proportional to the concentrations of the ions.
How solubilities relate to solubility products
The solubility (by which we usually mean the molar solubility) of a solid is expressed as the concentration of the "dissolved solid" in a saturated solution.
In the case of a simple 1:1 solid such as AgCl, this would just be the concentration of Ag+ or Cl– in the saturated solution. But for a more complicated stoichiometry such as as silver chromate, the solubility would be only one-half of the Ag+ concentration.
For example, let us denote the solubility of Ag2CrO4 as S mol L–1. Then for a saturated solution, we have
[Ag+] = 2S [CrO42–] = S.
Substituting this into the solubility product expression
Ag2CrO4(s) ![]() 2 Ag+ + CrO42–
2 Ag+ + CrO42–
yields
(2S)2 (S) = 4S3 = 2.76E–12
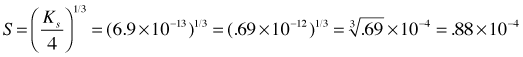 (5)
(5)
thus the solubility is 8.8 × 10–5 M.
Note that the relation between the solubility and the solubility product constant depends on the stoichiometry of the dissolution reaction. For this reason it is meaningless to compare the solubilities of two salts having the formulas A2B and AB2, say, on the basis of their Ks values.
In all honesty, I must point out that the simple relations between Ks and molar solubility outlined above, and the calculation examples given here, cannot be relied upon to give correct answers. Some of the reasons for this are explained in Part 2 of this lesson, and have mainly to do with incomplete dissociation of many salts and with complex formation in the presence of anions such as Cl– and OH–.
The situation is nicely described in the article What Should We Teach Beginners about Solubility and Solubility Products? by Stephen Hawkes (J Chem Educ. 1998 75(9) 1179-81). See also the earlier article by Meites, Pode and Thomas Are Solubilities and Solubility Products Related? (J Chem Educ. 1966 43(12) 667-72).
It turns out that solubility equilibria more often than not involve many competing processes and their rigorous treatment can be quite complicated. Unfortunately, most textbooks fail to mention this and many teachers are unaware of it.
Nevertheless, it is important that you master these over-simplified examples, but don't take them too seriously!
The common ion effect
It has long been known that the solubility of a sparingly soluble ionic substance is markedly decreased in a solution of another ionic compound when the two substances have an ion in common. This is just what would be expected on the basis of the LeChâtelier Principle; whenever the process
CaF2(s) ![]() Ca2+ + 2 F– (7)
Ca2+ + 2 F– (7)
is in equilibrium, addition of more fluoride ion (in the form of highly soluble NaF) will shift the composition to the left, reducing the concentration of Ca2+, and thus effectively reducing the solubility of the solid.
We can express this quantitatively by noting that the solubility product expression
[Ca2+][F–]2 = 1.7 × 10–10 (8)
must always hold, even if some of the ionic species involved come from sources other than CaF2(s). For example, if some quantity x of fluoride ion is added to a solution initially in equilibrium with solid CaF2, we have
[Ca2+] = S, [F–] = 2S + x
so that
Ks = [Ca2+][ F–]2 = S × (2S + x)2 . (9a)
In most practical cases x will be large compared to S so that the 2S term can be dropped and the relation becomes
Ks ≈ S x2 , S = Ks ÷ x2.(9b)
The plots shown below illustrate the common ion effect for silver chromate as the chromate ion concentration is increased by addition of a soluble chromate such as Na2CrO4.
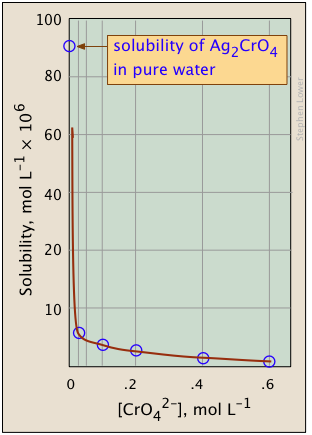
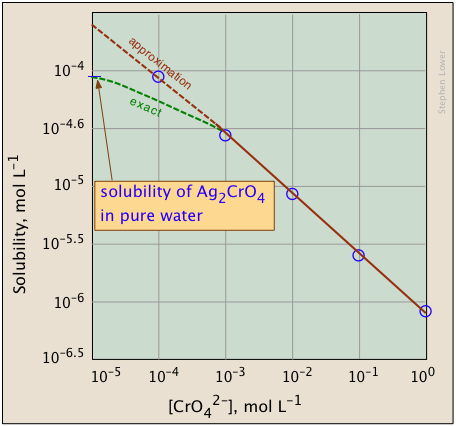
What's different about the plot on the right?
If you look carefully at the scales, you will see that this one is plotted logarithmically (that is, in powers of 10.)
Notice how a much wider a range of values can display on a logarithmic plot.
The point of showing this pair of plots is to illustrate the great utility of log-concentration plots in equilibrium calculations in which simple approximations (such as that made in Eq. 9b) can yield straight-lines within the range of values for which the approximation is valid.
How solubility differences can facilitate separations
Differences in solubility are widely used to selectively remove one species from a solution containing several kinds of ions.
Solubility equilibria defined by a single, simple Ks expression are probably the exception rather than the rule. Such equilibria are often in competition with other reactions with such species as H+or OH–, complexing agents, oxidation-reduction, formation of other sparingly soluble species or, in the case of carbonates and sulfites, of gaseous products. The exact treatments of these systems can be extremely complicated, involving the solution of large sets of simultaneous equations. For most practical purposes it is sufficient to recognize the general trends, and to carry out approximate calculations.
Why salts of weak acids dissolve in strong acids
The solubility of a sparingly soluble salt of a weak acid or base will depend on the pH of the solution. To understand the reason for this, consider a hypothetical salt MA which dissolves to form a cation M+ and an anion A– which is also the conjugate base of a weak acid HA. The fact that the acid is weak means that hydrogen ions (always present in aqueous solutions) and M+ cations will both be competing for the A–:

The weaker the acid HA, the more readily will reaction  take place, thus gobbling up A– ions. If an excess of H+ is made available by addition of a strong acid, even more A– ions will be consumed, eventually reversing reaction
take place, thus gobbling up A– ions. If an excess of H+ is made available by addition of a strong acid, even more A– ions will be consumed, eventually reversing reaction  , causing the solid to dissolve.
, causing the solid to dissolve.
... but strong acids will not dissolve salts of strong acids
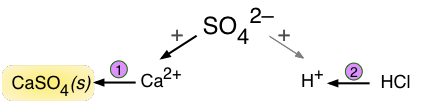
In  , for example, sulfate ions react with calcium ions to form insoluble CaSO4. Addition of a strong acid such as HCl (which is totally dissociated
, for example, sulfate ions react with calcium ions to form insoluble CaSO4. Addition of a strong acid such as HCl (which is totally dissociated  ) has no effect because CaCl2 is soluble. Although H+ can protonate some SO42– ions to form hydrogen sulfate ("bisulfate") HSO4–, this ampholyte acid is too weak to reverse
) has no effect because CaCl2 is soluble. Although H+ can protonate some SO42– ions to form hydrogen sulfate ("bisulfate") HSO4–, this ampholyte acid is too weak to reverse  by drawing a significant fraction of sulfate ions out of CaSO4(s).
by drawing a significant fraction of sulfate ions out of CaSO4(s).
Formation of a competing precipitate
If two different anions compete with a single cation to form two possible precipitates, the outcome depends not only on the solubilities of the two solids, but also on the concentrations of the relevant ions.
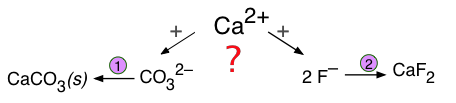
These kinds of competitions are especially important in groundwaters, which acquire solutes from various sources as they pass through sediment layers having different compositions. As the following example shows, competing equilibria of these kinds are very important for understanding geochemical processes involving the formation and transformation of mineral deposits.
Complex ion formation can compete with precipitation
If you are not familiar with complex ions, you might wish to see this overview of bonding and properties of coordination complexes.
Most transition metal ions possess empty d orbitals that are sufficiently low in energy to be able to accept electron pairs from electron donors from cations, resulting in the formation of a covalently-bound complex ion. Even neutral species that have a nonbonding electron pair can bind to ions in this way.
Water is an active electron donor of this kind, so aqueous solutions of ions such as Fe3+(aq) and Cu2+(aq) exist as the octahedral complexes Fe(H2O)63+ and Cu(H6O)62+, respectively.
Many of the remarks made above about the relation between Ks and solubility also apply to calculations involving complex formation. See Stephen Hawkes' article Complexation Calculations are Worse Than Useless ("... to the point of absurdity...and should not be taught" in introductory courses.) (J Chem Educ. 1999 76(8) 1099-1100)
But again, it is very important that you understand the principles outlined in this section.
H2O is only one possible electron donor; NH3, CN– and many other species (known collectively as ligands) possess lone pairs that can occupy vacant d orbitals on a metallic ion. Many of these bind much more tightly to the metal than does H2O, which will undergo displacement and substitution by one or more of these ligands if they are present in sufficiently high concentration.
If a sparingly soluble solid is placed in contact with a solution containing a ligand that can bind to the metal ion much more strongly than H2O, then formation of a complex ion will be favored and the solubility of the solid will be greater.
Perhaps the most commonly seen example of this occurs when ammonia is added to a solution of copper(II) nitrate, in which the Cu2+(aq) ion is itself the complex hexaaquo complex ion shown at the left:
to a solution of copper(II) nitrate, in which the Cu2+(aq) ion is itself the complex hexaaquo complex ion shown at the left:
Because ammonia is a weak base, the first thing we observe is formation of a cloudy precipitate of Cu(OH)2 in the blue solution. As more ammonia is added ![]() , this precipitate dissolves, and the solution turns an intense deep blue, which is the color of hexamminecopper(II) and the various other related species such as Cu(H2O)5(NH3)2+, Cu(H2O)4(NH3)22+, etc.
, this precipitate dissolves, and the solution turns an intense deep blue, which is the color of hexamminecopper(II) and the various other related species such as Cu(H2O)5(NH3)2+, Cu(H2O)4(NH3)22+, etc.
An illustrated discussion of ammonia complexes of other transition metal ions can be found on this UK ChemGuide page.

In many cases, the complexing agent and the anion of the sparingly soluble salt are identical. This is particularly apt to happen with insoluble chlorides, and it means that addition of chloride to precipitate a metallic ion such as Ag+ will produce a precipitate at first, but after excess Cl– has been added the precipitate will redissolve as complex ions are formed.


 image:
image: